Skip to main content
Logo image

Math in Society, 2nd Edition: Tools for decision making

Portland Community College Math Department

Appendix A Odd Answers

1 Logical Reasoning and Problem Solving
1.1 Logic in Everyday Life
1.1.11 Exercises

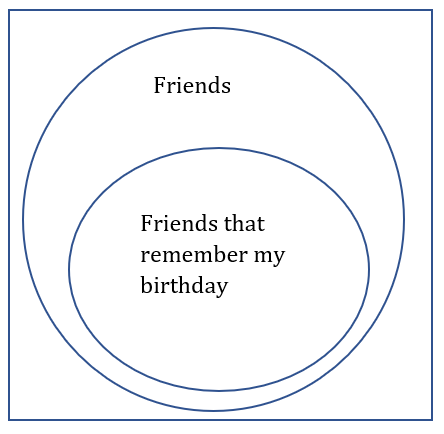
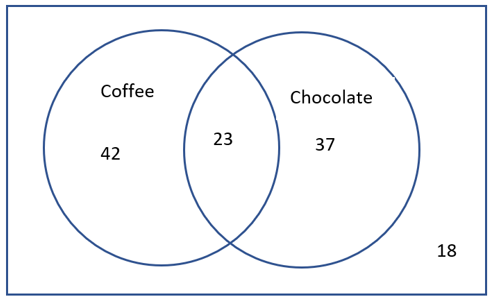
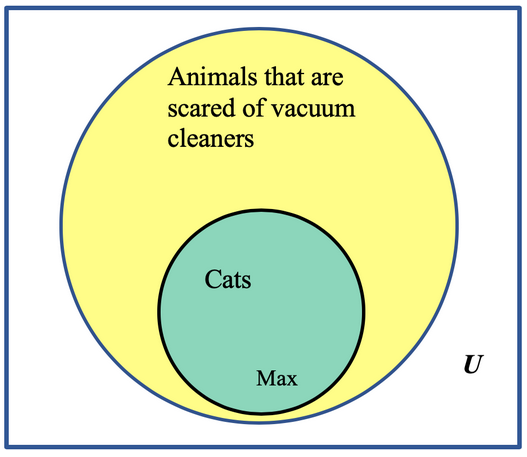
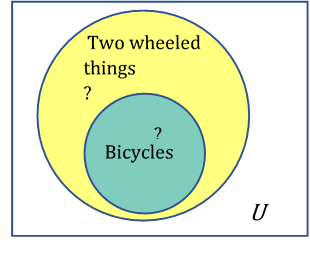
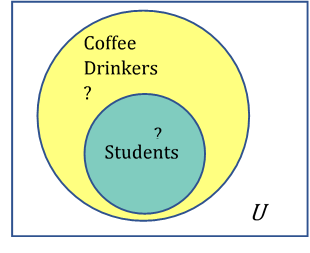
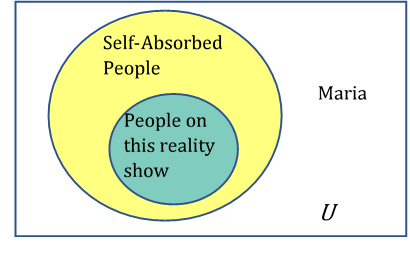
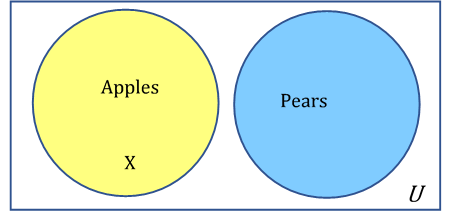
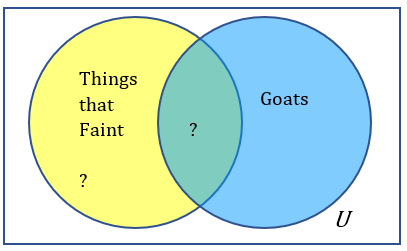
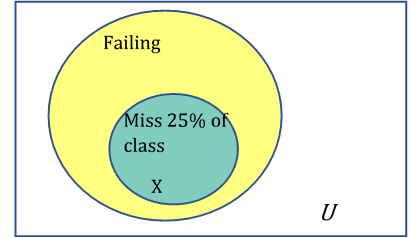
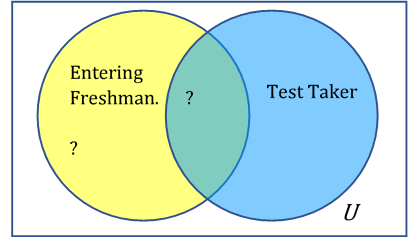
1.1.11.1.

Solution.
  1. Proposition
  2. Not a proposition
  3. Proposition
  4. Proposition

1.1.11.3.

Solution.
  1. I do not ride my bike to campus.
  2. Portland is in Oregon.

1.1.11.5.

Solution.
  1. Some people ride their bike to campus, or There are people who ride their bike to campus.
  2. There are cities named Portland that are not in Oregon (in Maine and Michigan), or Some Portlands are not in Oregon.

1.1.11.7.

Solution.
Answers will vary. Example: You can’t not do your homework.

1.1.11.9.

Solution.
  1. Exclusive
  2. Inclusive

1.1.11.11.

Solution.
  1. Inclusive
  2. Exclusive

1.1.11.13.

Solution.
  1. If it is sunny, then I will go swimming.
  2. If it is Friday, then I will go see a movie.

1.1.11.15.

Solution.
  1. True
  2. False
  3. False
  4. True

1.1.11.17.

Solution.
  1. True
  2. True
  3. False
  4. True

1.1.11.19.

Solution.
  1. Converse: If you have food for dinner then you did go to the store.
  2. Inverse: If you do not go to the store then you will not have food for dinner.
  3. Contrapositive: If you do not have food for dinner then you did not go to the store.

1.1.11.21.

Solution.
Statements i and iii are logically equivalent to each other. Statements ii and iv are logically equivalent to each other.

1.2 Sets and Venn Diagrams
1.2.12 Exercises

1.2.12.1.

Solution.
{m, i, s, p}

1.2.12.3.

Solution.
The set containing multiples of 3 from 3 to 9

1.2.12.5.

Solution.
Yes, {1, 3, 5} a subset of the set of odd numbers.

1.2.12.7.

Solution.

1.2.12.9.

Solution.
  1. Twenty-four students have seen exactly one of these movies.
  2. Nineteen students have only seen Star Wars Episode IX.

1.2.12.11.

Solution.
  1. Terriers and dogs
  2. Subsets

1.2.12.13.

Solution.
  1. Pigs and things that can fly
  2. Disjoint

1.2.12.15.

Solution.
  1. There are at least two ways this can be interpreted. Friends and people that remember your birthday OR friends and friends that remember my birthday.
  2. Overlapping or subset (depending on how this is interpreted)

1.2.12.17.

Solution.
  • Region A: Unemployed non-honor-students who identify as boys
  • Region B: Unemployed honor-students who identify as boys
  • Region C: Unemployed honor-students who identify as girls or nonbinary
  • Region D: Employed non-honor-students who identify as boys
  • Region E: Employed honor-students who identify as boys
  • Region F: Employed honor-students who identify as girls or nonbinary
  • Region G: Employed non-honor-students who identify as girls or nonbinary
  • Region H: Unemployed non-honor-students who identify as girls or nonbinary

1.2.12.19.

Solution.
  • Students enrolled for keyboard class: 24
  • Students enrolled for keyboard class only: 8
  • Students didn’t enroll at all: 6
  • Students took all three classes: 4
  • Students enrolled for guitar and drum: 6
  • Students enrolled for guitar and drum only: 2

1.2.12.21.

Solution.
  2. Nineteen said “It’s more filling!” but didn’t say “It tastes great!”
  3. Forty-two said neither of those things.
  4. 108 said “It’s more filling!” or said “It tastes great!”

1.3 Percents
1.3.8 Exercises

1.3.8.1.

Solution.
  1. 0.156
  2. 0.091
  3. 0.0007
  4. 1.356

1.3.8.3.

Solution.
  1. 89%
  2. 4.3%
  3. 75%
  4. 105%

1.3.8.5.

Solution.
Approximately 92.2% of the participants completed the race.

1.3.8.7.

Solution.
The tip is 18%.

1.3.8.9.

Solution.
Miguel will owe $2700 for the Portland Metro Tax.

1.3.8.11.

Solution.
About 280%.

1.3.8.13.

Solution.
About $81,850.

1.3.8.15.

Solution.
The tax is $10.44 and the total cost is $130.44.

1.3.8.17.

Solution.
Their energy use would need to be $1870 or less to have a decrease of 15%.

1.3.8.19.

Solution.
  • There was an absolute increase of 441 undergraduate students.
  • There was a relative increase of about 2.7%.

1.3.8.21.

Solution.
  • There was an absolute decrease of 90 students.
  • There was a relative decrease of about 2.7%.

1.3.8.23.

Solution.
A 100% increase.

1.3.8.25.

Solution.
A 20% decrease.

1.3.8.27.

Solution.
\(\$2,000,000(1-0.095)(1+.10)=\$1,991,000.\) for a relative decrease of 0.0045 or 0.45%.

1.3.8.29.

Solution.
\((1-0.60)(1-.30)=0.28\) or 28% of the original price.

1.4 Rates and Proportions
1.4.7 Exercises

1.4.7.1.

Solution.
  1. rate
  2. ratio
  3. unit rate
  4. 1.356

1.4.7.3.

Solution.
$0.50 per pound

1.4.7.5.

Solution.
The 9.6-ounce container is $0.565 per ounce and the 40.3-ounce canister is $0.369 per ounce. The larger container is a better value.

1.4.7.7.

Solution.
The population density of the US is about 83.4 people per square mile. The population density of India is about 93.4 people per square mile.

1.4.7.9.

Solution.
No, the second printer prints more pages per minute.

1.4.7.11.

Solution.
Yes, these rates are the same.

1.4.7.13.

Solution.
You would need 2 and a half cups of flour.

1.4.7.15.

Solution.
40 feet of crown molding would cost $70.

1.4.7.17.

Solution.
You would need at least 73.3 or 74 3-megawatt wind turbines.

1.4.7.19.

Solution.
You would need 9.6 or 10 packets of seeds.

1.4.7.21.

Solution.
You would get about $186.81.

1.4.7.23.

Solution.
For 5 scoops of mix you need 2.5 cups of milk. If there is no room in the cup pour it into a larger bowl and mix.

1.5 Problem Solving
1.5.6 Exercises

1.5.6.1.

Solution.
  1. Division.
  2. Addition and/or multiplication.

1.5.6.3.

Solution.
  1. Answers may vary. We estimate 300 to 500 steps.
  2. Answers may vary. We estimate 100 to 200 cans.

1.5.6.5.

Solution.
You will earn $760 this week.

1.5.6.7.

Solution.
Your profit is $750.

1.5.6.9.

Solution.
Your profit is $373.

1.5.6.11.

Solution.
You would need about 3.85 pounds of pecans.

1.5.6.13.

Solution.
The hourly job would pay $58,740 per year. The salary of $60,000 is higher unless there is a lot of overtime pay in the hourly job.

1.5.6.15.

Solution.
It would take 30 hours of overtime in a year for the hourly pay to equal the salaried job pay.

1.5.6.17.

Solution.
It depends on the size of the party. The per person venue is less expensive up to 52 people. The per table venue is cheaper for 53 or more guests.

1.5.6.19.

Solution.
You have 48+24=72 cups. You still need 28 cups.

1.5.6.21.

Solution.
The child should get approximately 7.5 mg per day. This would be 3 of the 2.5 mg tablets.

1.5.6.23.

Solution.
Parvina needs 27 bags of soil.

1.5.6.25.

Solution.
Answers will vary.

1.5.6.27.

Solution.
Answers will vary.

1.5.6.29.

Solution.
Answers will vary.

1.5.6.31.

Solution.
Answers will vary.

1.5.6.33.

Solution.
Answers will vary.

1.5.6.35.

Solution.
Answers will vary.

1.6 Chapter 1 Review

1.6.1.

Solution.
  1. Yes
  2. Yes
  3. No
  4. No
  5. Yes
  6. Yes

1.6.3.

Solution.
  1. Inclusive
  2. Inclusive
  3. Exclusive
  4. Exclusive
  5. Exclusive

1.6.5.

Solution.
  1. True
  2. True
  3. False
  4. False
  5. False

1.6.7.

Solution.
  1. \(27\) people only like cream in their coffee.
  2. \(13+20=33\)
    \(33\) people put sugar in their coffee
  3. \(27+13+20=60\)
    \(60\) people put sugar or cream in their coffee.
  4. \(20+10=30\)
    \(30\) people don’t like cream in their coffee.

1.6.9.

Solution.

1.6.11.

Solution.
There are 4.5 mg of iron in one serving of the cereal.

1.6.13.

Solution.
$70(2.5)(1-.30)=$122.50. The combined relative change is a markup of 175%

1.6.15.

Solution.
You would need 7.5 ounces of coffee.

1.6.17.

Solution.
We calculated 17 boxes of tile for a total cost of about $2158.

1.6.19.

Solution.
The child should get approximately 300 mg per day which is 10 ml per dose or 2 teaspoons.

1.7 Truth Tables
1.7.5 Exercises

1.7.5.1.

Solution.
  1. Elvis is not alive
  2. Elvis is alive or Elvis is the King
  3. Elvis is not alive and Elvis is the King
  4. If Elvis is the King, then Elvis is not alive

1.7.5.3.

Solution.
  1. B and A
  2. If B, then A
  3. If B, then A
  4. If not B, then not A

1.7.5.5.

Solution.
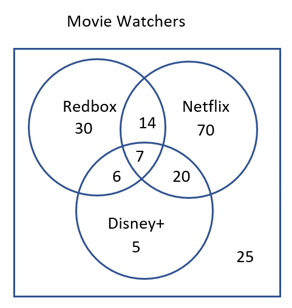
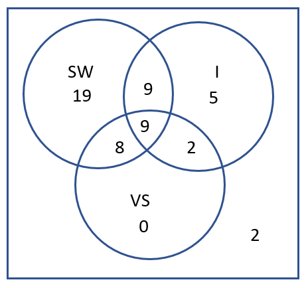
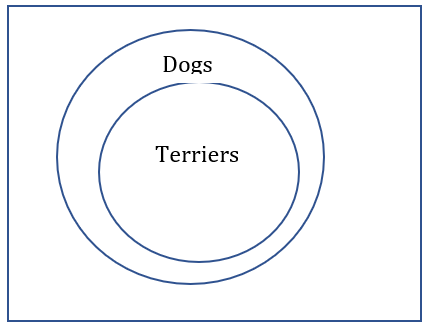
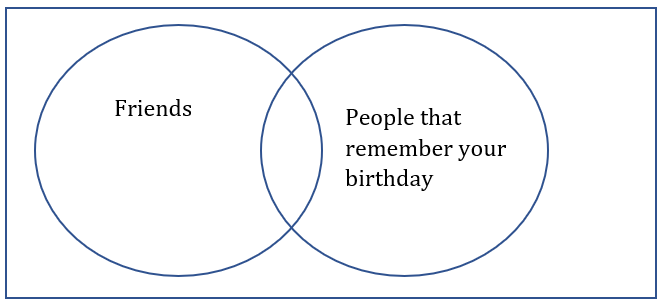
A B A and B
T T T - I live in Oregon and I go to PCC
T F F - I live in Oregon and I don’t go to PCC
F T F - I don’t live in Oregon and I go to PCC
F F F - I don’t live in Oregon and I don’t go to PCC

1.7.5.7.

Solution.
A B Not B A and not B
T T F F
T F T T
F T F F
F F T F

1.7.5.9.

Solution.
A B C A and B and C Not (A and B and C)
T T T T F
T T F F T
T F T F T
T F F F T
F T T F T
F T F F T
F F T F T
F F F F T

1.7.5.11.

Solution.
A B C A and B Not (A and B) Not (A and B) or C
T T T T F T
T T F T F F
T F T F T T
T F F F T T
F T T F T T
F T F F T T
F F T F T T
F F F F T T

1.7.5.13.

Solution.
A B C A and B If (A and B), then C
T T T T T
T T F T F
T F T F T
T F F F T
F T T F T
F T F F T
F F T F T
F F F F T

1.7.5.15.

Solution.
A C A and C Not A If (A and C), then not A
T T T F F
T F F F T
F T F T T
F F F T T

1.8 Describing and Critiquing Arguments
1.8.5 Exercises

1.8.5.1.

Solution.
  1. The argument is inductive.
  2. The argument is deductive.

1.8.5.3.

Solution.
Premise: All cats are scared of vacuum cleaners.
Premise: Max is a cat.
Conclusion: Max must be scared of vacuum cleaners.
This is a deductive argument. It is valid. However, it is not sound because the premise all cats are afraid of vacuum cleaners is false. While many cats are afraid of vacuum cleaners not ALL cats are afraid. There are many videos of cats riding electronic vacuum cleaners.

1.8.5.5.

Solution.
Premise: Kiran’s female and nonbinary friends made less than Kiran’s male friends.
Conclusion: Women and nonbinary people make less than men.
This is an inductive argument. Kiran did not gather a large diverse sample because they only asked their friends. Therefore, their data has sampling bias. This makes their argument weak.

1.8.5.7.

Solution.
Premise: All bicycles have two wheels.
Premise: My friend’s Harley-Davidson motorcycle has two wheels.
Conclusion: It must be a bicycle.
This is a deductive argument. The argument is not valid. Based on the premises, we know that the friend’s Harley-Davidson motorcycle has two wheels, but we do not know whethere or not it is a bicycle. Because it is not valid, it is also not sound.

1.8.5.9.

Solution.
Premise: All students drink a lot of caffeine.
Premise: Brayer drinks a lot of caffeine.
Conclusion: He must be a student.
This is a deductive argument. The argument is not valid. We can not determine if Brayer is a student or not. Because it is not valid it is also not sound.

1.8.5.11.

Solution.
Premise: People on this reality show are self-absorbed.
Premise: Laura is not self-absorbed.
Conclusion: Laura cannot be on this reality show.
This argument is deductive. The argument is valid because Laura is outside of the set of self-absorbed people, so she must also be outside of this set of people on this reality show. Determining if this conclusion is sound is more difficult because determining if someone is self-absorbed is subjective.

1.8.5.13.

Solution.
The argument is valid and sound.

1.8.5.15.

Solution.
The argument is not valid, and it is not sound.

1.8.5.17.

Solution.
This argument is valid, and it is sound. Because Ethan is in the set of folks who missed 25% of the classes he also falls into the set of failing.

1.8.5.19.

Solution.
This argument is not valid, and is not sound. We cannot determine if Juan took the test.

1.9 Logical Fallacies
1.9.9 Exercises

1.9.9.1.

Solution.
Personal attack

1.9.9.3.

Solution.
Personal Dilemma

1.9.9.5.

Solution.
Post Hoc

1.9.9.7.

Solution.
Personal Attack

1.9.9.9.

Solution.
Appeal to ignorance

1.9.9.11.

Solution.
False dilemma

1.9.9.13.

Solution.
Personal attack

1.9.9.15.

Solution.
Appeal to ignorance

1.9.9.17.

Solution.
Post Hoc

1.9.9.19.

Solution.
Personal Attack

2 Financial Math
2.1 Introduction to Spreadsheets
2.1.6 Exercises

2.1.6.1.

Solution.
=4/7 which gives approximately 0.571429

2.1.6.3.

Solution.
=8+19 which gives 27

2.1.6.5.

Solution.
=12*9 which gives 108

2.1.6.7.

Solution.
=8^3 which gives 512

2.1.6.9.

Solution.
=7.50/44.50 which gives approximately 0.1685, or approximately 16.85%

2.1.6.11.

Solution.
  1. =5780.23-5250 which gives $530.23
  2. =530.23/5250 which gives approximately 0.100996, or approximately 10.1%
  3. Exactly 200%
  4. =5250*115.5% which gives exactly $6063.75

2.1.6.13.

Solution.
  1. =1000*103%*103% which gives $1060.90
  2. =1000*(103%)^2 gives the same result of $1060.90, because raising 103% to the second power means the same as multiplying 103% by itself two times.
  3. =1000*(103%)^15 which gives $1557.97 rounded to the nearest cent
  4. Refer to the table at the bottom for part d and part e.
    Note the entry in cell B3 here is = B2*103% and the remaining cells are computed using the fill down feature.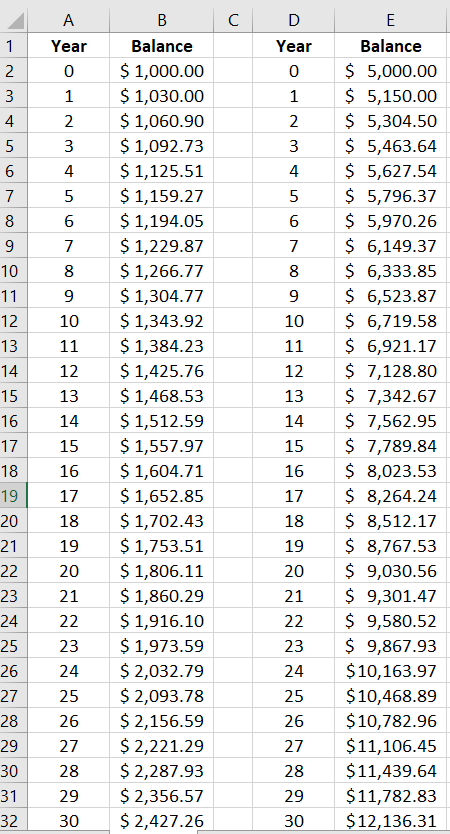
    You will have to wait a minimum of 24 full years, in each case, in order for the balance to finally exceed twice the opening deposit amount.
  5. Since \((103\%)^{23} \lt 2 \lt (103\%)^{24}\text{,}\) the minimum number of full years until the opening deposit doubles must be the same here, for any positive opening balance that we may choose for this account.
A portion of a spreadsheet is shown, which includes cells A1 through E32, and with a title heading of Table 3. Cell A1 shows a column title of Year, and cells A2 through A32 contain values of 0 through 30, respectively. Cells D1 through D32 are a copy of cells A1 through A32. Cell B1 shows a column title of Balance. Cell B2 shows $1,000.00, which corresponds to Year 0 displaying in adjacent cell A1. Cell B3 shows $1,030.00, which is 103% of the $1,000 shown in cell B2. Cell B4 shows $1,060.90, which is 103% of the $1,030.00 shown in cell B3. This pattern continues until we get to cell B32, which shows $2,427.26, and which is 103% of the $2,356.57 amount shown in cell B31. Cell E1 shows a column title of Balance. Cell B2 shows $5,000.00, which corresponds to Year 0 displaying in adjacent cell D1. Cell E3 shows $5,150.00, which is 103% of the $5,000 shown in cell E2. Cell E4 shows $5,304.50, which is 103% of the $5,150.00 shown in cell E3. This pattern continues until we get to cell E32, which shows $12,136.31, and which is 103% of the $11,782.83 amount shown in cell E31.

2.2 Simple and Compound Interest
2.2.11 Exercises

2.2.11.1.

Solution.
\begin{align*} A\amp=200+200(0.05)\\ \amp=\$210 \end{align*}
You will have to repay $210.

2.2.11.3.

Solution.
\begin{align*} A\amp=200+200(0.06)(1.25)\\ \amp=\$215 \end{align*}
$215 was repaid.

2.2.11.5.

Solution.
  1. \begin{align*} A\amp=20000+20000(0.05)(10)\\ \amp=\$30{,}000 \end{align*}
  2. \begin{align*} A\amp=20000(1+0.05)^{10}\\ \amp=\$32{,}577.89 \end{align*}
The simple interest account would be worth $30,000 and the account that was compounding would be worth $32,577.89 in ten years.

2.2.11.7.

Solution.
\begin{align*} A\amp=1000\left(1+\frac{0.07}{52}\right)^{52*20}\\ \amp\approx \$4{,}051.38 \end{align*}
The account balance is $4,051.38 in 20 years.
Or,
=FV(0.07/52,52*20,0,1000)

2.2.11.9.

Solution.
  1. \begin{align*} A\amp=300\left(1+\frac{0.05}{1}\right)^{1*10}\\ \amp\approx \$488.67 \end{align*}
    There will be $488.67 in the account in 10 years.
    Or,
    =FV(0.05/1,1*10,0,300)
  2. \begin{align*} I\amp=488.67-300\\ \amp=\$188.67 \end{align*}
    $188.67 of the balance will be interest.
  3. \begin{gather*} \frac{188.67}{488.67} \approx 0.3861 \text{ or } 38.61\% \end{gather*}
    The interest makes up 38.61% of the balance.

2.2.11.11.

Solution.
  1. \begin{align*} A\amp=10000\left(1+\frac{0.04}{52}\right)^{52*25}\\ \amp\approx \$27{,}172.37 \end{align*}
    The balance is $27,172.37.
    Or,
    =FV(0.04/52,52*25,0,10000)
  2. \begin{align*} I\amp=27{,}172.37-10{,}000\\ \amp=\$17{,}172.37 \end{align*}
    The interest is $17,172.37.
  3. \begin{gather*} \frac{17{,}172.37}{27{,}172.37} \approx 0.632 \rightarrow 63.2\% \end{gather*}
    The percent that is interest is 63.2%.
  4. \begin{gather*} \frac{10{,}000}{27{,}172.37} \approx 0.368 \rightarrow 36.8\% \end{gather*}
    The percentage that is the principal is 36.2%.

2.2.11.13.

Solution.
\begin{align*} P\amp=\frac{20000}{\left(1+\frac{0.05}{4}\right)^{4*4}}\\ \amp\approx \$16{,}394.79 \end{align*}
The principal required would be $16,394.79
Or,
=PV(0.05/4,4*4,0,20000)

2.2.11.15.

Solution.
  1. Bill =EFFECT(0.0375,12) \(=3.82\%\) and Ted =EFFECT(0.038,1) =3.8%. Bill has an effective rate of 3.82% and Ted has a rate of 3.8%.
  2. \begin{align*} A\amp=6700\left(1+\frac{0.0375}{12}\right)^{12*5}\\ \amp\approx \$8{,}079.38 \end{align*}
    Or, =FV(0.0375/12,5*12,0,6700)
    \begin{align*} A\amp=6500\left(1+\frac{0.038}{1}\right)^{1*5}\\ \amp\approx \$7{,}832.49 \end{align*}
    Or, =FV(0.038,5,0,6500)
    The account balances are $8,079.38 and $7,832.49. So, Bill’s balance is higher.

2.2.11.17.

Solution.
  1. \begin{align*} A\amp=2500e^{0.04*10}\\ \amp\approx \$3{,}729.56 \end{align*}
    Or, 2500*EXP(0.04*10)
    The account balance is $3,729.56.
  2. \begin{align*} I \amp= 3{,}729.56-2{,}500\\ \amp= \$1{,}229.56 \end{align*}
    The interest earned is $1,229.56.
  3. \begin{gather*} \frac{1{,}229.56}{3{,}729.56} \approx 0.3297 \rightarrow 32.97\% \end{gather*}
    32.97% of the balance is interest.

2.2.11.19.

Solution.
  1. \begin{align*} A\amp= 5000e^{0.045*5}\\ \amp\approx \$6{,}261.61 \end{align*}
    Or, =5000*EXP(0.045*5)
    The account balance is $6,261.61.
  2. \begin{align*} I \amp= 6{,}261.61-5{,}000\\ \amp= \$1{,}261.61 \end{align*}
    The interest earned is $1,261.61
  3. \begin{gather*} \frac{1{,}261.61}{6{,}261.61} \approx 0.2015 \rightarrow 20.15\% \end{gather*}
    The interest is 20.15% of the balance.

2.3 Savings Plans
2.3.5 Exercises

2.3.5.1.

Solution.
  1. \(\frac{250[(1+\frac{0.065}{12})^{12*35}-1]}{(\frac{0.065}{12})}\)
    Or, FV(0.065/12,12*35,250)
    In 35 years you will have $400,079.05 in your retirement plan.
  2. \(400{,}079.05 – 12*35*250\text{.}\) You will have earned $295,079.05 in interest.
  3. \(29{,}5079.05 /400{,}079.05\text{.}\) The final balance will be about 73.8% interest.

2.3.5.3.

Solution.
  1. \(\frac{750\left(1+\frac{0.0775}{4})^{4*30}-1\right)}{\left(frac{0.0775}{4}\right)}\)
    Or, =FV(0.0775/4,4*30,750)
    In 30 years you will have $348,456.10 in your retirement plan.
  2. \(348{,}456.1 – 4*30*750\text{.}\) You will have earned $258,456.10 in interest.
  3. \(258{,}456.1 /348{,}456.1\text{.}\) The final balance will be about 74.2% interest.

2.3.5.5.

Solution.
  1. In 5 years: \(\frac{130\left(\left(1+\frac{0.09}{12}\right)^{12*5}-1\right)}{\left(\frac{0.09}{12}\right)}\)
    Or, =FV(0.09/12,12*5,130)
    In 25 more years: \(9805.14(1+\frac{0.09}{12})^{12*25}\)
    =FV(0.09/12,12*25,0,9805.14)
    Your final balance will be $92,250.82.
  2. \(92{,}250.82 – 130*5*12\text{.}\) You will earn $84,450.82 in interest.
  3. \(84{,}450.82/92{,}250.82\text{.}\) The final balance will be about 91.5% interest.

2.3.5.7.

Solution.
\(\frac{3500\left(\frac{0.038}{12}\right)}{\left(1+\frac{0.038}{12}\right)^{30}-1}\)
=PMT(0.038/12,30,0,3500)
You should deposit $111.40 each month.

2.3.5.9.

Solution.
\(\frac{450000\left(\frac{0.06}{12}\right)}{\left(1+\frac{0.06}{12}\right)^{12*30}-1}\)
Or, =PMT(0.06/12,12*30,0,450000)
Jamie needs to deposit $447.98 each month.

2.3.5.11.

Solution.
  1. Jose: \(55000\left(1+\frac{0.056}{12}\right)^{12*25}\)
    Or, =FV(0.056/12,12*25,0,55000)
    Jose’s partner:\(\frac{375\left(\left(1+\frac{0.056}{12}\right)^{12*25}-1\right)}{\left(\frac{0.056}{12}\right)}\)
    Or, =FV(0.056/12,12*25,375)
    Jose will have $222,310.85 and his partner will have $244,447.68.
  2. Jose: \(222{,}310.85 – 55{,}000\)
    Jose’s partner: \(24{,}4447.68 – 375*12*25\)
    Jose will earn $167,310.85 and his partner will earn $131,947.68 in interest.
  3. Jose: \(167{,}310.85/222{,}310.85\)
    Jose’s partner: \(13{,}1947.68/244{,}447.68\)
    Jose’s final balance will be about 75.3% interest and Jose’s partner’s final balance will be about 54.0% interest.

2.3.5.13.

Solution.
  1. \(1000\left(1+\frac{0.045}{12}\right)^{12*10}+\frac{100\left(\left(1+\frac{0.045}{12}\right)^{12*10}-1\right)}{\frac{0.045}{12}}\)
    Or, =FV(0.045/12,12*10,100,1000)
    Sylvin will have a final balance of $16,686.80.
  2. \(16{,}686.8 – (1000 + 100*12*10)\text{.}\) Sylvin will earn $3,686.80 in interest.
  3. \(3{,}686.8/16{,}686.8\text{.}\) The final balance will be about 22.1% interest.

2.3.5.15.

Solution.
  1. \(\frac{100\left(\left(1+\frac{0.04}{12}\right)^{12*25}-1\right)}{\frac{0.04}{12}}\)
    Or, =FV(0.04/12,12*25,100)
    Vanessa will have $51,412.95 when she turns 65.
  2. \(\frac{100\left(\left(1+\frac{0.04}{12}\right)^{12*40}-1\right)}{\frac{0.04}{12}}\)
    =FV(0.04/12,12*40,100
    Vanessa would have $118,196.13 if she had started saving when she was 25.

2.3.5.17.

Solution.
  1. \(\frac{50\left(\left(1+\frac{0.035}{52}\right)^{52*18}-1\right)}{\frac{0.035}{52}}\)
    Or, =FV(0.035/52,52*18,50)
    They will have saved $65,164.37 after 18 years.
  2. \(\frac{65164.37}{(1+\frac{0.035}{52})^{52*18}}\)
    Or, =PV(0.035/52,52*18,0,65164.37)
    They would have needed an deposit of $34,713.37.

2.4 Loan Payments
2.4.9 Exercises

2.4.9.1.

Solution.
  1. \(P = \frac{700\left(1-\left(1+\frac{5.5\%}{12}\right)^{-12\times 30}\right)}{\frac{5.5\%}{12}}\text{,}\) which gives \(P \approx \$123{,}285.23\)
    or =PV(0.055/12,12*30,-700) [Note 700 is entered as negative, to signify a payment]
  2. \(700 * 12 * 30\) dollars, or $252,000.00 in total payments to the loan company
  3. Interest will be the difference between the total payments, and the amount borrowed. So the interest on this loan is \(\$252{,}000.00 - \$123{,}285.23 = \$128{,}714.77\text{.}\)

2.4.9.3.

Solution.
\(d = \frac{25000\left(\frac{2\%}{12}\right)}{\left(1-\left(1+\frac{2\%}{12}\right)^{-12 \times 4}\right)}\text{,}\) which gives \(d \approx \$542.38\)
or =PMT(0.02/12,48,25000)

2.4.9.5.

Solution.
  1. The loan amount will be 90% of $200,000.00
    \begin{gather*} = (0.9 * \$200{,}000.00)\\ = \$180{,}000.00 \end{gather*}
  2. \(d = \frac{180000\left(\frac{5\%}{12}\right)}{\left(1-\left(1+\frac{5\%}{12}\right)^{-12 \times 30}\right)}\text{,}\) which gives \(d \approx \$966.28\)
    or =PMT(0.05/12,12*30,180000)
  3. \(d = \frac{180000\left(\frac{6\%}{12}\right)}{\left(1-\left(1+\frac{6\%}{12}\right)^{-12 \times 30}\right)}\text{,}\) which gives \(d \approx \$1{,}079.19\)
    or =PMT(0.06/12,12*30,180000)

2.4.9.7.

Solution.
First, we need to find out the amount of the monthly payments for this loan.
\(d = \frac{24000\left(\frac{3\%}{12}\right)}{\left(1-\left(1+\frac{3\%}{12}\right)^{-12 \times 5}\right)}\text{,}\) which gives \(d \approx \$431.25\)
or =PMT(0.03/12,12*5,24000)
The amount still owed three years later, is the present value of the two years of remaining payments on the loan.
\(P = \frac{\left(1-\left(1+\frac{3\%}{12}\right)^{-12 \times 2}\right)}{\frac{3\%}{12}}\text{,}\) which gives \(P \approx \$10{,}033.45\)
or =PV(0.03/12,12*2,-431.25)

2.4.9.9.

Solution.
=PV(0.04/12,12*30,950) which gives $198,988.18.
Now add $20,000 to this balance, which gives $218,988.18.

2.4.9.11.

Solution.
  1. =PMT(0.05,10,100000,0) which gives $12,950.46.
  2. The total payments will be $129,504.60.
    The interest is the amount over the $100,000 initial investment, or $29,504.60.
    The percentage of the total payment sum representing interest will be \(100(29,504.60/129,504.60)\%\text{,}\) or approximately 22.7827%.

2.4.9.13.

Solution.
  1. =PMT(0.045/12,12*30,250000) which gives $1,266.71.
  2. This will be the present value of the remaining 240 loan payments:
    =PV(0.045/12,12*20,1266.71) which gives $200,223.07.
  3. This will be the present value of the remaining 120 loan payments:
    =PV(0.045/12,12*10,1266.71) which gives $122,223.99.
  4. At the beginning of the repayment period, most of each payment goes to interest (thus the loan balance reduces very slowly at first). Over time, more of each payment shifts to principal, and less to interest. At the end of the loan repayment period, nearly all the payment is going to principal.

2.4.9.15.

Solution.
=FV(0.035/12,3*12,200,0) = $7,579.95. Paul will have $7,579.95 in 3 years.

2.4.9.17.

Solution.
=PV(0.028/12,4*12,100,0) = $4,535.96. Miao can finance $4,535.96 in equipment to have a monthly loan payment of $100 for 4 years.

2.4.9.19.

Solution.
=PMT(0.043/12,30*12,364500,0) = $1,803.81. Their mortgage payment would be $1,803.81.

2.4.9.21.

Solution.
For the first plan: =FV(0.048/12,20*12,150,0) = $60,251.26. Zahid would have $60,251.26 if he puts in $150 per month for 20 years.
For the second plan: =FV(0.048/12,10*12,300,0) = $46,089.59. Zahid would only have $46,089.59 if he waited and put in $300 per month for 10 years.

2.5 Income Taxes
2.5.14 Exercises

2.5.14.1.

Solution.
A credit decreases your bill more. It decreases your bill by the full amount of the credit. A deduction only decreases your tax bill by a percentage.

2.5.14.3.

Solution.
The amount of taxes owed is decreased by $60 because 12% of $500 is $60.

2.5.14.5.

Solution.
Yes, you can make adjustments and take a deduction. Adjustments to your income happen before deductions.

2.5.14.7.

Solution.
Shaysiah should itemize because itemizing reduces her taxable income by $15,400. The standard deduction would have reduced her taxable income by $14,600.

2.5.14.9.

Solution.
No, Fredrick should not be concerned. Only the $1000 will be taxed at 22%. The rest of his income will be taxed at a lower level.

2.5.14.11.

Solution.
There is a total of $35,550 between $11,600 and $47,150. 12% of $35,550 is $4,266.

2.5.14.13.

Solution.
$16,589 in taxes minus $13,456 for withholdings, and they can claim and $2,500 in credits. This leaves them owing $633.

2.5.14.15.

Solution.
  1. Take the income minus the adjustments \(135000-5600=\$129{,}400\text{.}\) Their adjusted gross income is $129,400.
  2. They should take the standard deduction because itemizing saves them less.
  3. Taxable income: \(129400-29200=100200\)
    Taxes owed: \(10852+0.22*(100200-94300)=\$12{,}150\)
    They owe $12,150 in taxes.
  4. \(12150-15000=-\$2{,}850\) Take the taxes owed minus credits and withholdings. They will receive a refund for $2,850.

2.5.14.17.

Solution.
  1. Gross Income: \(96000+850 = \$96{,}850\)
  2. Adjusted Gross Income: \(96850-26000 = \$70{,}850\)
  3. She should take itemized deductions since they are greater than the standard deduction for head of household.
  4. Taxable Income: \(70850-22600 = \$48{,}250 \)
  5. Tax from Table: \(1655 + 0.12*(48250 - 16550) = \$5{,}459\text{.}\) Owed/Refund: \(5459 – 4000 – 5300 = - \$3{,}841\)
    Janice will receive a refund of $3,841.

2.6 Chapter 2 Review

2.6.1.

Solution.
  1. \(I=1525(0.056)(14)\)
    \(A=1525+1525(0.056)(14)\)
    The interest is $1,195.6 and balance is $2,720.6.
  2. \(A=1525(1+\frac{0.056}{4})^{4\cdot14}\)
    Or, =FV(0.056/4, 4*14, 0, 1525)
    The interest is $1,796.97 and the balance is $3,321.97.
  3. \(A=1525(1+\frac{0.056}{52})^{52\cdot14}\)
    Or, =FV(0.056/52, 52*14, 0, 1525)
    The interest is $1,813.67 and the balance is $3,338.67.
  4. \(A=1525e^{0.056\cdot14}\)
    Or, =1525*EXP(0.056*14)
    The interest is $1,815.08 and the balance is $3,340.08.

2.6.3.

Solution.
\(A=25000(1+\frac{0.065}{4})^{4\cdot15}\)
\(I=65761.77-25000=40761.77\)
Or, =FV(0.065/4, 4*15, 0, 25000)
The interest is $40,761.77 and the amount paid is $65,761.77.

2.6.5.

Solution.
\(P=3500-350=\$3150\)
\(I=3150(0.13)(2.33) \approx \$955.50\)
You would pay $4,105.50 in 28 months with $146.63 as your monthly payments.

2.6.7.

Solution.
  1. \(A= \frac{350((1+\frac{0.065}{12})^{12\cdot25}-1)}{\frac{0.065}{12}}\)
    Or, =FV(0.065/12, 12*25, 350, 0)
    The future value is $262,092.78 and the interest earned is $157,092.78.
  2. \(A= \frac{500((1+\frac{0.065}{4})^{4\cdot15}-1)}{\frac{0.065}{4}}\)
    Or, =FV(0.065/4, 4*15, 500, 0)
    The future value is $50,168.34 and the interest earned is $20,168.34.
  3. \(A= \frac{75((1+\frac{0.045}{52})^{52\cdot30}-1)}{\frac{0.045}{52}}\)
    Or, =FV(0.045/52, 52*30, 75, 0)
    The future value is $247,448.43 and the interest earned is $130,448.43.

2.6.9.

Solution.
The net monthly cash flow is $428.

2.6.11.

Solution.
\(1000(0.12) = 120\text{.}\) Your tax bill will be decreased by $120.

2.6.13.

Solution.
  1. \(43,000+1,000 = 44,000\text{.}\) Amir’s gross income is $44,000.
  2. \(44,000-3,000=41000\text{.}\) Amir’s adjusted gross income is $41,000.

2.6.15.

Solution.
\(2936-1200-2500=-764\text{.}\) Amir will be refunded $764.

3 Statistics
3.1 Overview of the Statistical Process
3.1.22 Exercises

3.1.22.1.

Solution.
A sample is a sub group of the population. A population is the entire group of subjects.

3.1.22.3.

Solution.
The intended population is all PCC students.
  1. All PCC Students
  2. 200 students from PCC Cascade campus
  3. The collected data is not representative of all PCC students since it only includes responses from students at Cascade campus. This is an example of sampling bias.

3.1.22.5.

Solution.
  1. The representatives in a state’s congress.
  2. The population size is \(n=106\)
  3. The sample size is in \(n=28\)
  4. The statistic is \(\frac{14}{28}=0.5\) or 50%
  5. The confidence interval is \((45\%, 55\%)\) and tells us that the true percentage of the state congress representatives in support of the new education (the parameter) likely lies between 45% and 55%.

3.1.22.7.

Solution.
The population is all trout in the lake. The sample is the 20 that were caught.

3.1.22.9.

Solution.
Parameter

3.1.22.11.

Solution.
Statistic

3.1.22.13.

Solution.
  1. Stratified
  2. Volunteer
  3. Simple Random Sample

3.1.22.15.

Solution.
  1. Loaded Question
  2. Volunteer Bias
  3. Response Bias
  4. Volunteer
  5. Response Bias
  6. Response Vias or Non-response Bias

3.1.22.17.

Solution.
  1. Observational study
  2. Experiment
  3. Observational study

3.1.22.19.

Solution.
  1. Cancer patients
  2. No because sampling has variability
  3. Stratified
  4. Convenient Sample. It does not represent the population.

3.1.22.21.

Solution.
  1. All students
  2. Experiment
  3. It is only looking at one class and not all groups that are in the population so Subjects are not randomly sampled from a specified population.

3.1.22.23.

Solution.
  1. 0.05 or 5%
  2. (25%, 35%)
  3. I am confident that the percentage of college freshmen who prefer morning classes is between 25% to 35%.

3.1.22.25.

Solution.
  1. \((24+36)/2=30\text{.}\) The statistic is 30%.
  2. \(30-24=6\text{.}\) The margin of error is 6%.

3.1.22.27.

Solution.
Play Barry Manilow to half the crop and don’t play any music to the other half of the crop.

3.1.22.29.

Solution.
Answers will vary depending on the article.

3.2 Describing Data
3.2.24 Exercises

3.2.24.1.

Solution.
True

3.2.24.3.

Solution.
  1. Quantitative
  2. Categorical
  3. Categorical
  4. Quantitative
  5. Quantitative

3.2.24.5.

Solution.
Bar graphs and pie charts are used for categorical data.

3.2.24.7.

Solution.
  1. 2 had 3 children.
  2. 15 adults were questioned.
  3. 33.33% of the adults questioned had 0 children.

3.2.24.9.

Solution.
5 students earned and A on their paper.

3.2.24.11.

Solution.
Cory spent $676 on rent this month.

3.2.24.13.

Solution.
  1. These data are categorical.
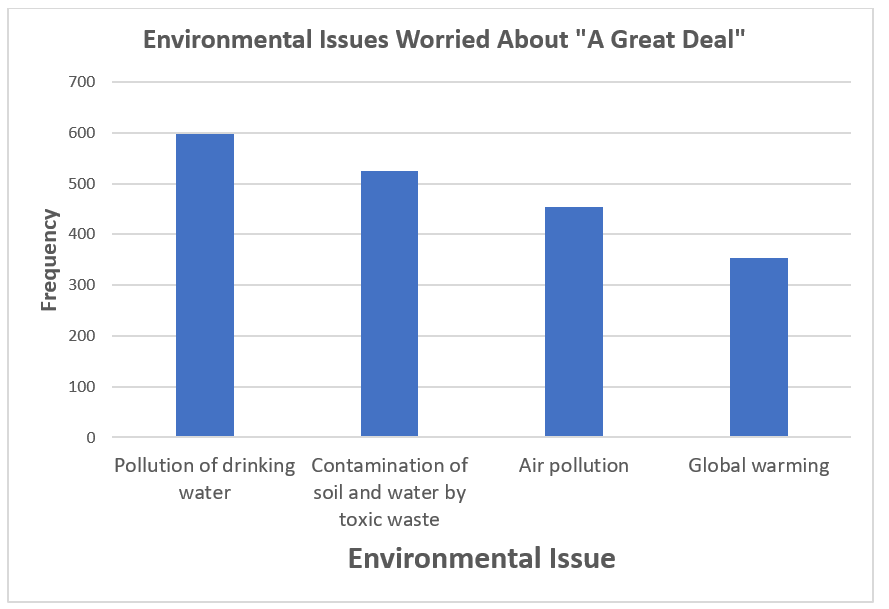
  2. There are four spaced apart bars labeled Pollution of drinking water, Contamination of soil and water by toxic waste, Air pollution, and Global warming. The x-axis is labeled Environmental Issue and the y-axis is labeled frequncy. Pollution of drinking water goes up to 597, Contamination of soil and drinking water goes up to 526, Air pollution goes up to 455, and Global warming goes up to 354.
  3. No, we cannot make a pie chart out of these data. The total of the relative frequencies is 1932, but only 1012 adults were asked. So some adults selected multiple options.

3.2.24.15.

Solution.
  1. 40 households heat their home with firewood.
  2. 50% of the households heat their home with natural gas.

3.2.24.17.

Solution.
  1. These data are qualitative.
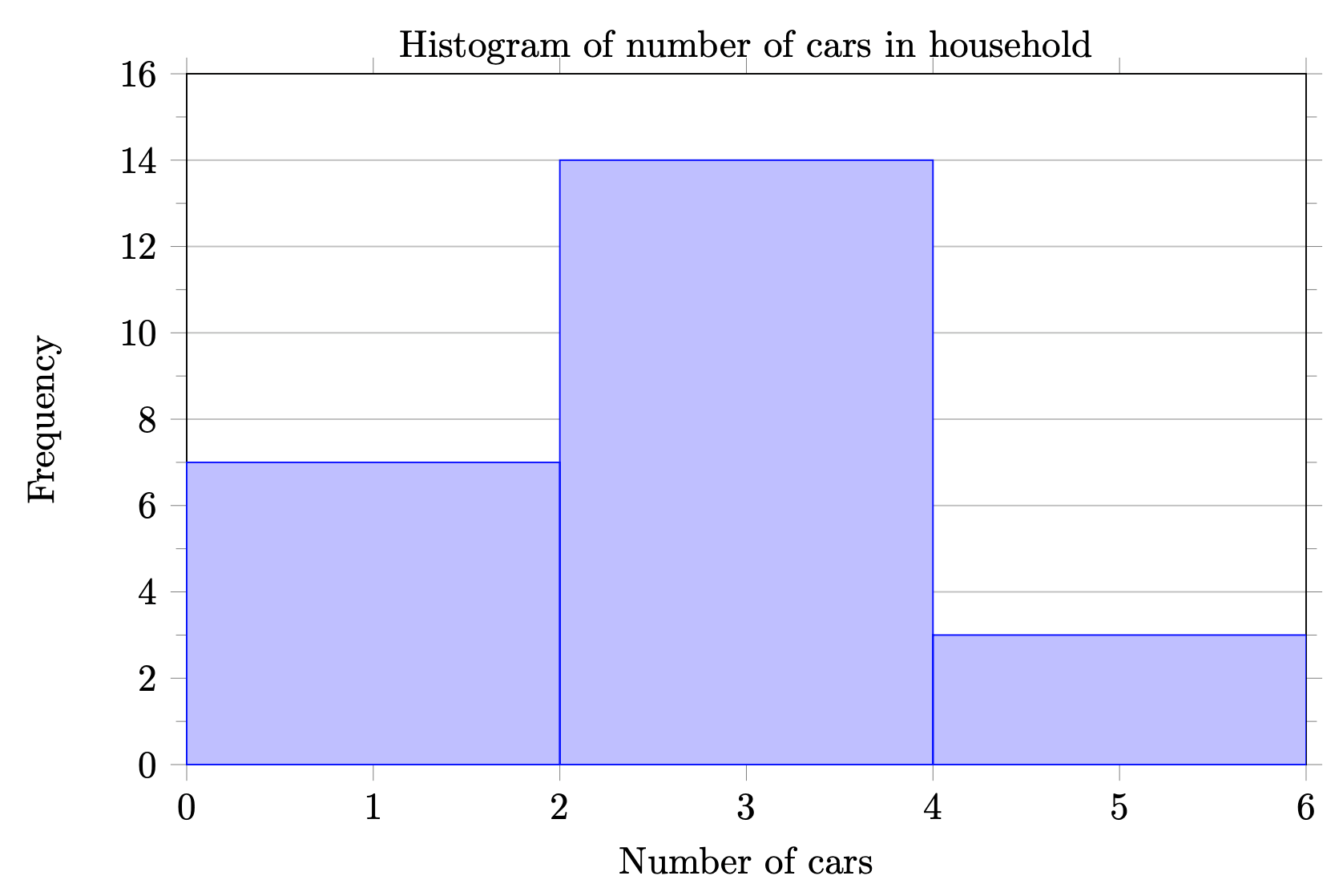
  2. Number of cars
    in household    		 Frequency
    0-1 7
    2-3 14
    4-5 3
  3. The histogram is titled "Histogram of number of cars in household". The x-axis is labeled Number of Cars, the scale goes from 0 to 6 and jumps by 1 unit. There are three bars, one from 0-2, one from 2-4, and one from 4-6. The y-axis is labeled Frequency and the scale goes from 0 to 16 by units of two. The 0-2 bar goes to 7, the 2-4 bar goes to 14, and the 4-6 bar goes to 3.
  4. These data are unimodal and skewed right, with no outliers.

3.2.24.19.

Solution.
The graph would be more effective at displaying the true differences between the categories if the vertical scale started at 0. The vertical axis is missing a label and units, so we can’t tell if those are frequencies or relative frequencies. A flat bar graph (instead of the 3d graph) would be easier to read.

3.2.24.21.

Solution.
This is a poor graph because the vertical axis does not have a numerical scale, so we cannot know how many have each drink as a favorite drink. We also don’t know if the bottom vertical line represents 0, which is potentially misleading.

3.2.24.23.

Solution.
  1. Normal distribution – The number of heads in 24 sets of 100 coin flips.
  2. Positive or right skewed – Distribution of scores on a psychology test.
  3. Negative or left skewed – Scores on a 20-point statistics quiz.
  4. Bimodal – The frequency of times between eruptions of the Old Faithful geyser.

3.2.24.25.

Solution.
  2. The data for patients of both researchers are symmetric. Researcher’s 1 patients’ data appears to be unimodal, but Researcher 2’s patients’ data may be bimodal or multimodal. The data for Researcher 1’s patients does not have any outliers, but the data for Researcher’s 2 may have outliers between 0 and 5 months or 40 and 45 months.

3.3 Summary Statistics: Measures of Center
3.3.7 Exercises

3.3.7.1.

Solution.
  1. In Excel:
    =average(7.50,25,10,10,7.50,8.25,9,5,15,8,7.25,7.50,8,7,12)
    \(=\$9.80\)
    There are 15 amounts shown, so \(n=15\text{.}\) The mean is:
    \begin{align*} \bar{x} \amp= \frac{(7.50+25+10+10+7.50+8.25+9+5+15+8+7.25+7.50+8+7+12)}{15}\\ \amp=\$9.80 \end{align*}
  2. In Excel:
    =median(7.50,25,10,10,7.50,8.25,9,5,15,8,7.25,7.50,8,7,12)
    \(=\$8.00\)
    There are 15 times shown, so \(n=15\text{.}\) We start by listing the data in order:
    $5, $7, $7.25, $7.50, $7.50, $7.50, $8, $8, $8.25, $9, $10, $10, $12, $15, $25
    \(Median=\$8.00\)
  3. Since the mean is greater than the median, we would expect the distribution will be skewed right.

3.3.7.3.

Solution.
  1. In Excel:
    =average(15.2,18.8,19.3,19.7,20.2,21.8,22.1,29.4)
    \(=20.81\) seconds
    There are 8 times shown, so \(n=8\text{.}\)
    \begin{align*} \bar{x} \amp= \frac{(15.2+18.8+19.3+19.7+20.2+21.8+22.1+29.4)}{15}\\ \amp=20.81 \text{ seconds} \end{align*}
  2. In Excel:
    =median(15.2,18.8,19.3,19.7,20.2,21.8,22.1,29.4)
    \(=19.95\) seconds
    There are 8 times shown, so \(n=8\text{.}\) The times are given already in order:
    15.2, 18.8, 19.3, 19.7, 20.2, 21.8, 22.1, 29.4
    \begin{align*} Median\amp=\frac{19.7+20.2}{2}\\ \amp=19.95 \text{ seconds} \end{align*}
  3. Since the mean and median are approximately equal, we would expect that the distribution is symmetric.

3.3.7.5.

Solution.
  1. In GeoGebra Classic, enter the costs into the column A and frequencies into column B of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option.
    \begin{gather*} Mean=33.8 \text{ thousand dollars} \end{gather*}
    The sum of the frequencies is 75, so \(n=75\)
    \begin{align*} \bar{x}\amp=\frac{15\cdot 3+20\cdot 7+25\cdot 10+30\cdot 15+35\cdot 13+40\cdot 11+45\cdot 9+50\cdot 7}{75}\\ \amp=33.8 \text{ thousand dollars} \end{align*}
  2. In GeoGebra Classic, enter the costs into the column A and frequencies into column B of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option.
    \begin{gather*} Median=35 \text{ thousand dollars} \end{gather*}
    Since there are 75 values (an odd number), we know that the median will be the single middle data value. Because \(\frac{75}{2}=37.5\text{,}\) we know it will be the 38th value in the list. The 38th value is 35, so the median is 35 thousand dollars.
  3. Since the mean is less than the median, we would expect the distribution to be skewed left.

3.3.7.7.

Solution.
  1. For Researcher 1:
    In Excel:
    =average(A1:A40)
    \(=23.6\) months.
    =median(A1:A40)
    \(=24\) months
    The mean for Researcher 1’s patients is 23.6 months, and the median for Researcher’s 1 patients is 24 months.
    For Researcher 2:
    In Excel:
    =average(A1:A40)
    \(=22.8\) months
    =median(A1:A40)
    \(=22\) months
    The mean for Researcher 2’s patients is 22.8 months, and the median is 22 months
  2. Both the mean and median for Researcher 1’s patients are greater than the mean and median for Researcher 2’s patients. So, on average, Researcher 1’s patients have a longer life time after starting the cancer treatment than Researcher 2’s patients.

3.3.7.9.

Solution.
GeoGebra was used to create the histograms. You should check with your instructor to see if histograms are to be hand-drawn or computer generated. Answers will vary depending on the size of the margins and the programs you are using.
  1. Figure 3.3.16. Histogram for Average Number of Pieces Correctly Remembered by Non-players
    Figure 3.3.17. Histogram for Average Number of Pieces Correctly Remembered by Beginners
    Figure 3.3.18. Histogram for Average Number of Pieces Correctly Remembered by Tournament Players
  2. The mean number of pieces correctly remembered for non-players was 33.65 pieces.
    The mean number of pieces correctly remembered for beginners was 47.6 pieces.
    The mean number of pieces correctly remembered for tournament players was 64.98 pieces.
  3. The median number of pieces correctly remembered for non-players was 33.5 pieces.
    The median number of pieces correctly remembered for beginners was 51.3 pieces.
    The median number of pieces correctly remembered for tournament players was 71.1 pieces.
  4. The distribution for non-players appears to be uniform. The distribution for beginners looks unimodal and left-skewed. The distribution for tournament players appears bimodal and symmetric.
    The mean and median number of pieces correctly remembered were both greatest for tournament players, with non-players having the smallest mean and median of pieces correctly remembered.

3.3.7.11.

Solution.
  1. There are many possible answers for this problem. Three data sets with 5 values each that have the same mean but different medians are:
    0, 0, 0, 0, 10
    0, 0, 2, 4, 4
    0, 1, 1, 1, 7
  2. There are many possible answers for this problem. Three data sets with 5 values that have the same median but different means are:
    10, 10, 10, 10, 10
    0, 0, 10, 15, 20
    1, 5, 10, 10, 10

3.3.7.13.

Solution.
  1. This graph is skewed left.
  2. I expect that the mean is less than the median because the graph is skewed left.

3.3.7.15.

Solution.
  1. Incomes are skewed to the right so the mean would be greater than the median.
  2. Weights are approximately symmetric so the mean would be about the same as the median.
  3. Number of children is skewed to the right so the mean would be greater than the median.
  4. Medical costs for all adults are most likely skewed to the right so the mean would be greater than the median.
  5. Medical costs for adults 65+ may be symmetric or skewed to the right. Answer according to the shape you chose.

3.4 Summary Statistics: Measures of Variation
3.4.10 Exercises

3.4.10.1.

Solution.
  1. In Excel:
    Entering the data values into cells A1 through A15.
    \begin{align*} s\amp=stdev.s(A1:A15)\\ \amp=\$4.82 \end{align*}
    From Exercise 3.3.7.1, the mean is $9.80. There are 15 data values, so \(n=15\text{.}\)
    We will make a table of data values, their deviations from the mean, and the squared deviations:
    Data Value Deviation Deviation Squared
    \(7.5\) \(7.5-9.8=-2.3\) \((-2.3)^2=5.29\)
    \(25\) \(25-9.8=15.2\) \((15.2)^2=231.04\)
    \(10\) \(10-9.8=0.2\) \((0.2)^2=0.04\)
    \(10\) \(10-9.8=0.2\) \((0.2)^2=0.04\)
    \(7.5\) \(7.5-9.8=-2.3\) \((-2.3)^2=5.29\)
    \(8.25\) \(8.25-9.8=-1.55\) \((-1.55)^2=2.4\)
    \(9\) \(9-9.8=-0.8\) \((-0.8)^2=0.64\)
    \(5\) \(5-9.8=-4.8\) \((-4.8)^2=23.04\)
    \(15\) \(15-9.8=5.2\) \((5.2)^2=27.04\)
    \(8\) \(8-9.8=-1.8\) \((-1.8)^2=3.24\)
    \(7.25\) \(7.25-9.8=-2.55\) \((-2.55)^2=6.5\)
    \(7.5\) \(7.5-9.8=-2.3\) \((-2.3)^2=5.29\)
    \(8\) \(8-9.8=-1.8\) \((-1.8)^2=3.24\)
    \(7\) \(7-9.8=-2.8\) \((-2.8)^2=7.84\)
    \(12\) \(12-9.8=2.2\) \((2.2)^2=4.84\)
    Next, we add the squared deviations and get \(5.29 + 231.04 + 0.04 + 0.04 + 5.29 + 2.4 + 0.64 + 23.04 + 27.04 + 3.24 + 6.5 + 5.29 + 3.24 + 7.84 + 4.84 = 325.78\) dollars-squared.
    The sample standard deviation is:
    \begin{align*} s\amp=\sqrt{\frac{325.78}{14}}\\ \amp=\$4.82 \end{align*}
  2. In GeoGebra:
    In GeoGebra Classic, enter the data values into the column A of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option to find the five-number summary.
    Min Q1 Median Q3 Max
    $5 $7.50 $8 $10 $25
    From Exercise 3.3.7.1, the data listed in order is:
    $5.00, $7.00, $7.25, $7.50, $7.50, $7.50, $8.00, $8.00, $8.25, $9.00, $10.00, $10.00, $12.00, $15.00, $25.00
    Also from Exercise 3.3.7.1, there are 15 data values (\(n=15\)), and the median is $8.00. The lower half of the data is:
    $5.00, $7.00, $7.25, $7.50, $7.50, $7.50, $8.00
    The median of the lower half is $7.50, so the lower quartile \(Q_{1}\) is $7.50.
    The upper half of the data is:
    $8.25, $9.00, $10.00, $10.00, $12.00, $15.00, $25.00
    The median of the upper half is $10.00, so the upper quartile \(Q_{3}\) is $10.00.
    The smallest and largest data values are $5.00 and 25.00, respectively, so the min and max are $5.00 and $25.00. The five-number summary is:
    Min Q1 Median Q3 Max
    $5 $7.50 $8 $10 $25
  3. The range is:
    \begin{align*} Range \amp= Max - Min\\ \amp=25-5\\ \amp=\$20 \end{align*}
    The interquartile range (IQR) is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=10-7.5\\ \amp=\$2.50 \end{align*}

3.4.10.3.

Solution.
  1. In Excel:
    I entered the data values into cells A1 through A9.
    The standard deviation is:
    \begin{align*} s\amp=stdev.s(A1:A9)\\ \amp=4.068 \text{ seconds} \end{align*}
  2. In GeoGebra:
    In GeoGebra Classic, enter the data values into the column A of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option to find the five-number summary.
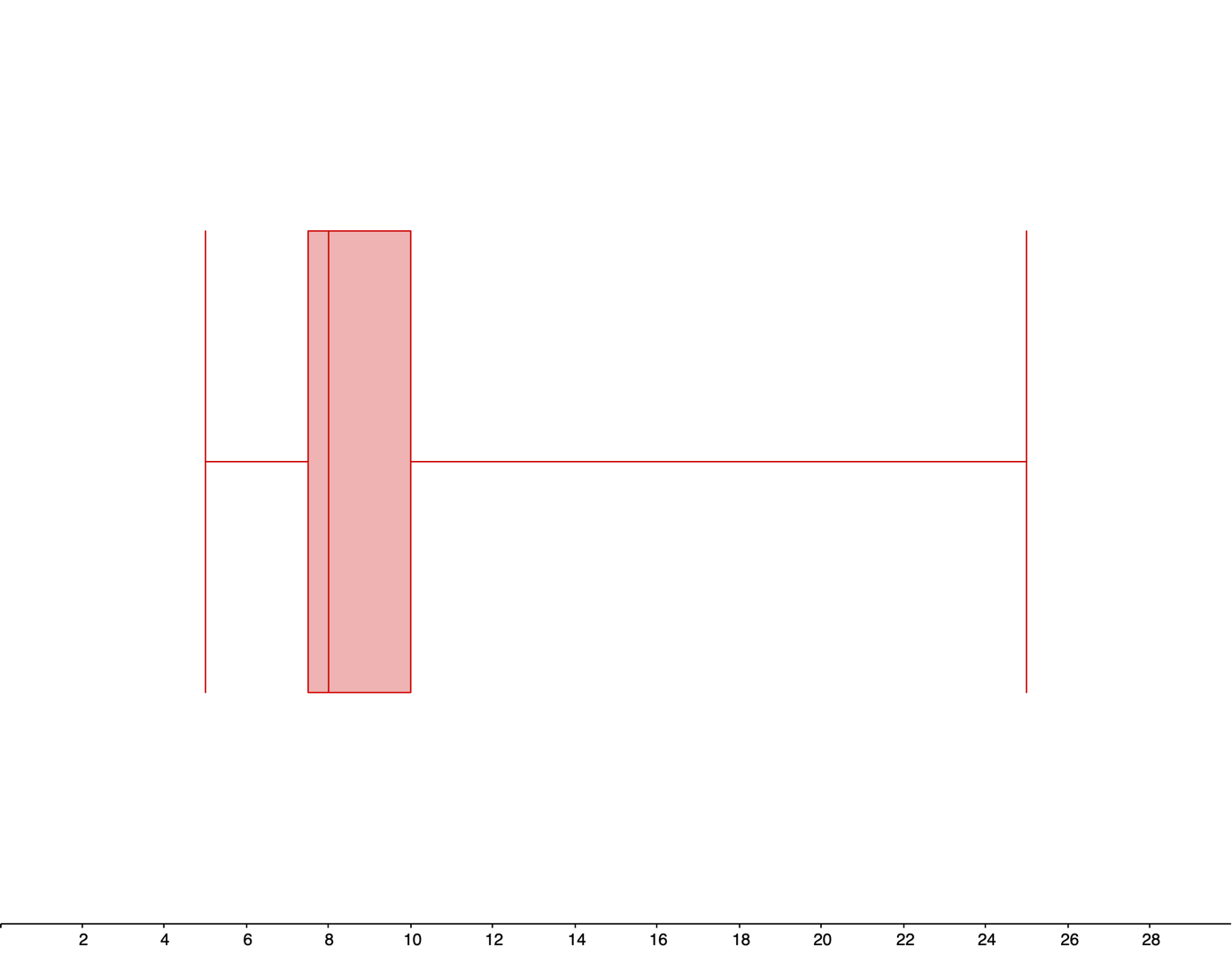
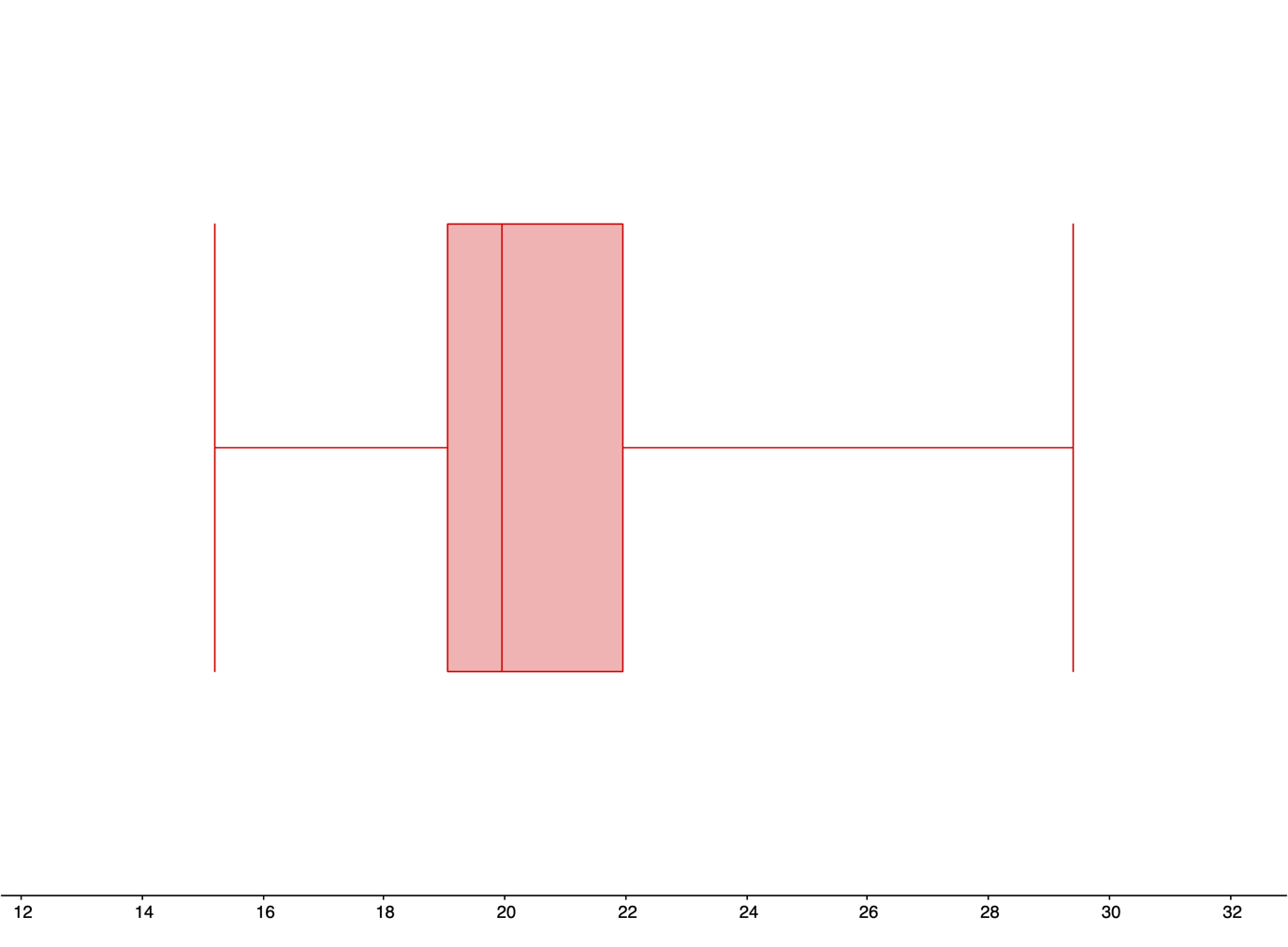
    Min Q1 Median Q3 Max
    15.2 seconds 19.05 seconds 19.95 seconds 21.95 seconds 29.4 seconds
  3. The range is:
    \begin{align*} Range \amp= Max - Min\\ \amp=29.4-15.2\\ \amp=14.2 \text{ seconds} \end{align*}
    The interquartile range (IQR) is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=21.95-19.05\\ \amp=2.9 \text{ seconds} \end{align*}

3.4.10.5.

Solution.
  1. In GeoGebra:
    In GeoGebra Classic, enter the costs into column A and frequencies into column B of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option. The standard deviation is:
    \begin{gather*} s=9.58 \text{ thousand dollars} \end{gather*}
    From Exercise 3.3.7.5, the mean is 33.8 thousand dollars.
    The mean and the standaed deviation together tell us that, on average, the cars at the local dealership are $9,580 from the mean price of $33,800.
  2. In GeoGebra:
    In GeoGebra Classic, enter the data values into the column A of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option to find the five-number summary.
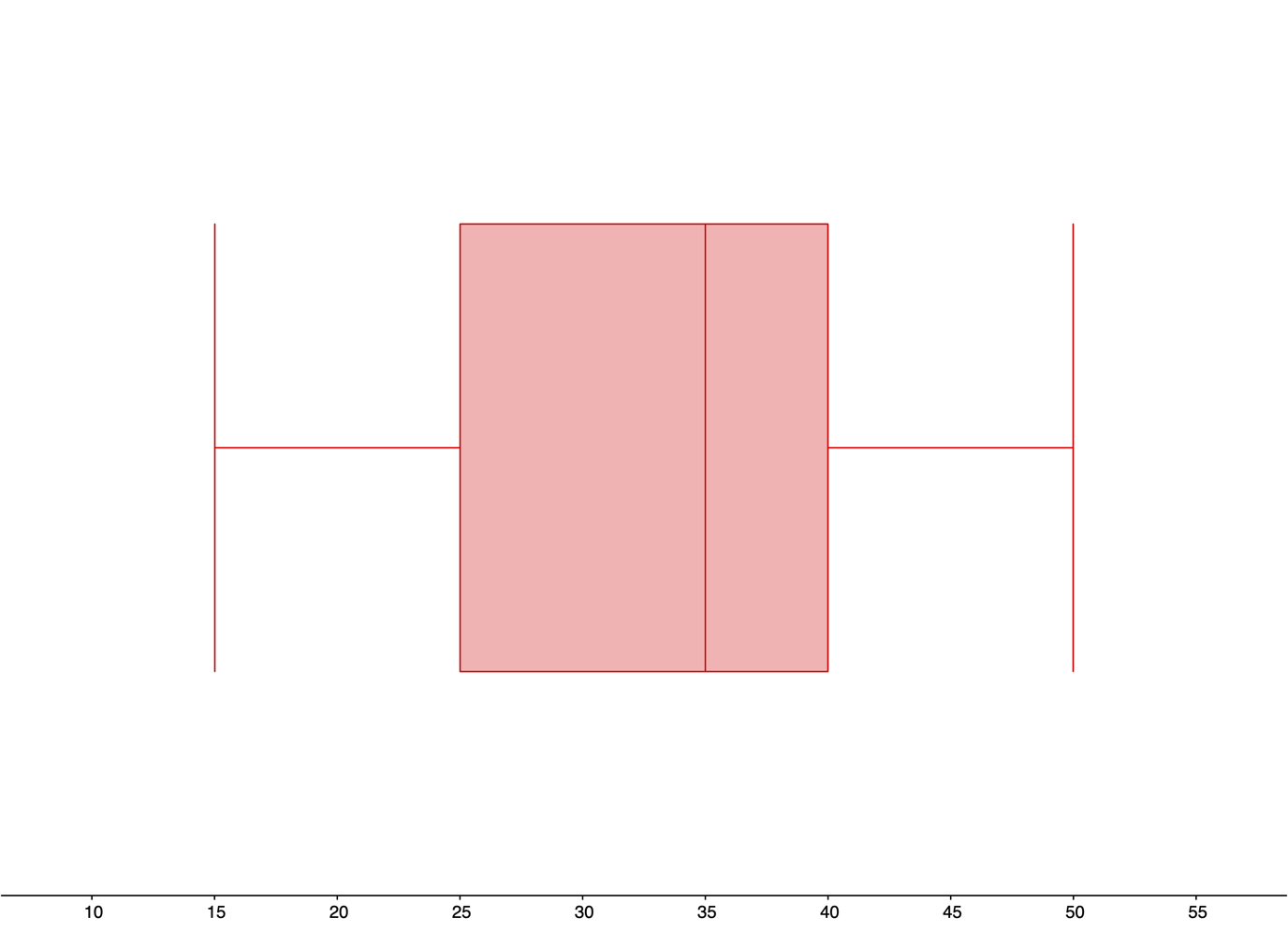
    Min Q1 Median Q3 Max
    15 thousand
    dollars    		 25 thousand
    dollars    		 35 thousand
    dollars    		 40 thousand
    dollars    		 50 thousand
    dollars
  3. The range is:
    \begin{align*} Range \amp= Max - Min\\ \amp=50-15\\ \amp=35 \text{ thousand dollars} \end{align*}
    The interquartile range (IQR) is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=40-15\\ \amp=25 \text{ thousand dollars} \end{align*}

3.4.10.7.

Solution.
  1. In GeoGebra:
    In GeoGebra Classic, enter the data values for Researcher 1 into column A of the spreadsheet, and enter the data values for Researcher 2 into column B of the spreadsheet. Then use the “Multiple Variable Analysis” function. Then use the “Show Statistics” function to display the sample standard deviation for each set of data values.
    The sample standard deviation for Researcher 1 is 11.25 months. The sample standard deviation for Research 2 is 11.38 months.
  2. In GeoGebra:
    In GeoGebra Classic, enter the data values for Researcher 1 into column A of the spreadsheet, and enter the data values for Researcher 2 into column B of the spreadsheet. Then use the “Multiple Variable Analysis” function. Then use the “Show Statistics” function to display the sample standard deviation for each set of data values.
    The 5-number summary for Researcher 1 is:
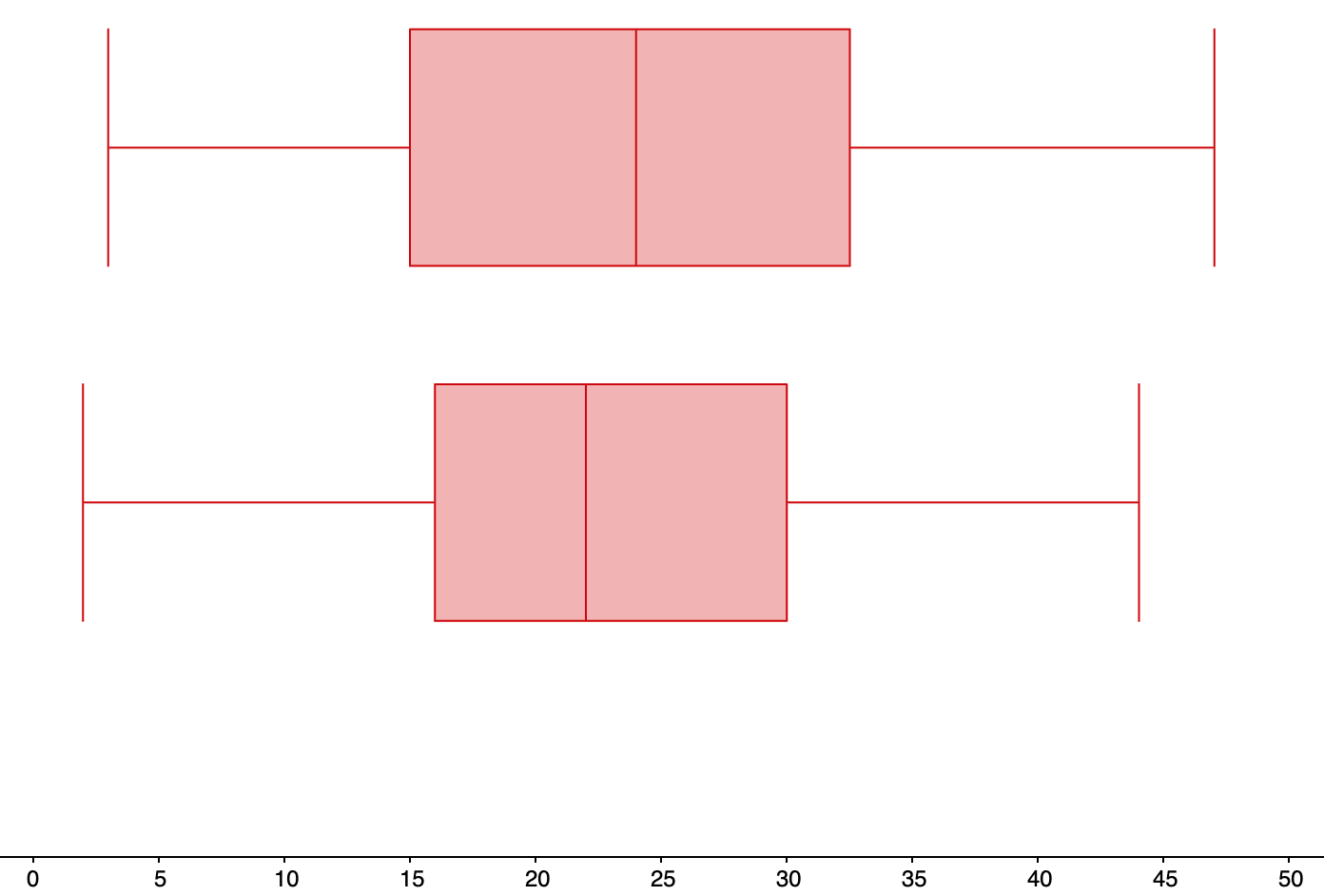
    Min Q1 Median Q3 Max
    3 months 15 months 24 months 32.5 months 47 months
    The 5-number summary for Researcher 2 is:
    Min Q1 Median Q3 Max
    2 months 16 months 22 months 30 months 44 months
  3. The range for Researcher 1 is:
    \begin{align*} Range \amp= Max - Min\\ \amp=47-3\\ \amp=44 \text{ months} \end{align*}
    The interquartile range (IQR) for researcher 1 is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=32.5-15\\ \amp=17.5 \text{ months} \end{align*}
    The range for Researcher 2 is:
    \begin{align*} Range \amp= Max - Min\\ \amp=44-2\\ \amp=42 \text{ months} \end{align*}
    The interquartile range (IQR) for Researcher 2 is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=30-16\\ \amp=14 \text{ months} \end{align*}
  4. In GeoGebra Classic, enter the data values for Researcher 1 into column A of the spreadsheet, and enter the data values for Research 2 into column B of the spreadsheet. Then use the “Multiple Variable Analysis” function. Then select “Stacked BoxPlots” from the drop-down menu.
    Researcher 1 has a larger minimum, median, 3rd quartile, and maximum than Researcher 2. For Researcher 1, 50% of the patients live longer than 24 months after treatment, compared to 50% of patients living longer than 22 months after treatment for Researcher 1.
    Researcher 2 has less variation in the life times than Researcher 1, with an IQR of 14 months for Researcher 2, compared to an IQR 16.5 months for Researcher 1.

3.4.10.9.

Solution.
  1. In GeoGebra:
    In GeoGebra Classic, enter the data values for non-players into column A of the spreadsheet, enter the data values for beginners into column B of the spreadsheet, and enter the data value for tournament players into column C of the spreadsheet. Then use the “Multiple Variable Analysis” function. Then use the “Show Statistics” function to display the sample standard deviation for each set of data values.
    The sample standard deviation for non-players is 8.033 chess pieces. The sample standard deviation for beginners is 9.031 chess pieces. The sample standard deviation for tournament players is 15.622 chess pieces.
  2. In GeoGebra:
    In GeoGebra Classic, enter the data values for non-players into column A of the spreadsheet, enter the data values for beginners into column B of the spreadsheet, and enter the data value for tournament players into column C of the spreadsheet. Then use the “Multiple Variable Analysis” function. Then use the “Show Statistics” function to display the sample standard deviation for each set of data values.
    The 5-number summary for non-players is:
    Min Q1 Median Q3 Max
    22.1 chess
    pieces    		 26.2 chess
    pieces    		 32.6 chess
    pieces    		 39.7 chess
    pieces    		 43.2 chess
    pieces
    The 5-number summary for beginners is:
    Min Q1 Median Q3 Max
    32.5 chess
    pieces    		 39.1 chess
    pieces    		 48.4 chess
    pieces    		 55.7 chess
    pieces    		 57.7 chess
    pieces
    The 5-number summary for tournament palyers is:
    Min Q1 Median Q3 Max
    40.1 chess
    pieces    		 51.2 chess
    pieces    		 64.6 chess
    pieces    		 75.9 chess
    pieces    		 85.3 chess
    pieces
  3. The range for non-players is:
    \begin{align*} Range \amp= Max - Min\\ \amp=43.2-22.1\\ \amp=21.2 \text{ chess pieces} \end{align*}
    The interquartile range (IQR) for non-players is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=39.7-26.2\\ \amp=13.5 \text{ chess pieces} \end{align*}
    The range for beginners is:
    \begin{align*} Range \amp= Max - Min\\ \amp=57.7-32.5\\ \amp=25.2 \text{ chess pieces} \end{align*}
    The interquartile range (IQR) for beginners is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=55.7-39.1\\ \amp=16.6 \text{ chess pieces} \end{align*}
    The range tournament players for is:
    \begin{align*} Range \amp= Max - Min\\ \amp=85.3-40.1\\ \amp=45.2 \text{ chess pieces} \end{align*}
    The interquartile range (IQR) for tournament players is:
    \begin{align*} IQR \amp= Q_{3} - Q_{1}\\ \amp=75.9-51.2\\ \amp=24.7 \text{ chess pieces} \end{align*}
  4. Tournament players did the best at remembering positions (as shown by all of the numbers of their 5-number summary being larger than the corresponding numbers for the other two groups). However, tournaments players were not completely superior to the other two groups; the best non-players remembered more chess pieces than the worst tournament players. Also tournament players had more variation in how much they.

3.4.10.11.

Solution.
  1. There are many possible answers for this question. For example, the data sets {10, 10, 10, 10, 10} and {9, 9, 10, 11, 11} have the same mean of 10 units, but different standard deviations (0 and 1, respectively).
  2. There are many possible answers for this question. For example, the data sets {2, 2, 2, 2, 2} and {9, 9, 9, 9, 9} have the same standard deviation of 0, but different means (2 and 9, respectively).

3.4.10.13.

Solution.
  1. The 25th, 50th, and 75th percentiles are, respectively, the 1st quartile, median, and 3rd quartile for the data sets. Reading the boxplot for CPAs, the 25th, 50th, and 75th percentiles for CPAs’ salaries are, respectively, $40,000, $75,000, and $90,000. Reading the boxplot for actuaries, the 25th, 50th, and 75th percentiles for actuaries’ salaries are, respectively, $75,000, $90,000, and $94,000
  2. Deshawn’s salary (the median salary for an actuary) is $90,000; Kelsey’s salary (the first quartile salary) is also $75,000. So Deshawn makes more than Kelsey, by $15,000.
  3. 75% of actuaries make more than the median salary of a CPA ($75,000).
  4. 25% of all CPAs earn less than all actuaries.

3.4.10.15.

Solution.
  1. \begin{align*} Z\amp=\frac{\text{data value}-\text{mean}}{\text{standard deviation}}\\ \amp=\frac{21.4-25}{1.15}\\ \amp=-3.13 \text{ standard deviations} \end{align*}
  2. The \(Z\)-score for the gas mileage of the car is -3.13 standard deviations.

3.4.10.17.

Solution.
  1. In GeoGebra:
    In GeoGebra Classic, enter the data values into the column A of the spreadsheet and use the “One Variable Analysis” function. Then use the “Show Statistics” option to find the mean and standard deviation.
    The mean is 46.2 hours per year, and the standard deviation is 6.16 hours per year.
  2. \begin{align*} Z\amp=\frac{\text{data value}-\text{mean}}{\text{standard deviation}}\\ \amp=\frac{42-46.2}{6.16}\\ \amp=-0.68 \text{ standard deviations} \end{align*}
    The \(Z\)-score for a city with an average delay time of 42 hours per year is -0.68 standard deviations.

3.4.10.19.

Solution.
\begin{align*} Z_{Math}\amp=\frac{\text{data value}-\text{mean}}{\text{standard deviation}}\\ \amp=\frac{89-75}{7}\\ \amp=2 \text{ standard deviations} \end{align*}
\begin{align*} Z_{English}\amp=\frac{\text{data value}-\text{mean}}{\text{standard deviation}}\\ \amp=\frac{65-53}{4}\\ \amp=3 \text{ standard deviations} \end{align*}
Because the \(Z\)-score of my English test is greater than the \(Z\)-score of my math test, I did better on the English test than I did on the math test.

3.4.10.21.

Solution.
\begin{align*} Z_{Poe}\amp=\frac{\text{data value}-\text{mean}}{\text{standard deviation}}\\ \amp=\frac{20.2-16.5}{1.85}\\ \amp=2 \text{ standard deviations} \end{align*}
\begin{align*} Z_{Gibson}\amp=\frac{\text{data value}-\text{mean}}{\text{standard deviation}}\\ \amp=\frac{107-81}{13}\\ \amp=2 \text{ standard deviations} \end{align*}
Because the \(Z\)-scores for the heights of Poe (the Clydesdale horse) and Gibson (the Great Dane) are the same, neither animal is taller than the other when compared to their respective breeds.

3.5 Chapter 3 Review

3.5.1.

Solution.
  1. The population is PCC students and 73,000 is the sample size.
  2. The sample is 250 students from the 4 main campuses.
  3. Stratified Sample
  4. Categorical or Qualitative
  5. \(\displaystyle 435/1000 = 0.435 = 43.5\%\)

3.5.3.

Solution.
  1. The population being studied is PCC Students.
  2. Categorical or Qualitative
  3. 23% is a statistic because it is from a sample.
  4. The margin of error is 4%.
  5. \(23\% - 4\% = 19\%\) and \(23\% + 4\% = 27\%\text{.}\) The confidence interval is \((19\%, 27\%)\text{.}\)
  6. We are confident that the true proportion of all PCC students who prefer to study at the library is between 19% and 27%

3.5.5.

Solution.
  1. Voluntary Response Bias
  2. Sampling Bias
  3. Sampling Bias
  4. Response bias
  5. Perceived lack of anonymity

3.5.7.

Solution.
  1. The treatment group is the group receiving the test medicine for migraines.
  2. The control group is the group receiving the inert pill.
  3. This is a blind study.
  4. This is a placebo-controlled experiment.

3.5.9.

Solution.
  1. The implied population is United States teenagers.
  2. There were 2007 teens surveyed.
  3. Qualitative or Categorical
  6. Answers will vary
  7. About 14.3% of teens chose horror as their favorite movie genre.

3.5.11.

Solution.
  1. The mean is $4.78 per gallon. The median is $4.75 per gallon.
  2. The mean and median is about the same value therefore the data is symmetric.
  3. The standard deviation is $1.34 per gallon.
  4. \(z_{\$3.25}=\frac{\$3.25-\$4.78}{\$1.34}=\frac{-\$1.53}{\$1.34}=-1.14\)
    \(z_{\$8.95}=\frac{\$8.95-\$4.78}{\$1.34}=\frac{\$4.17}{\$1.34}=3.11\)
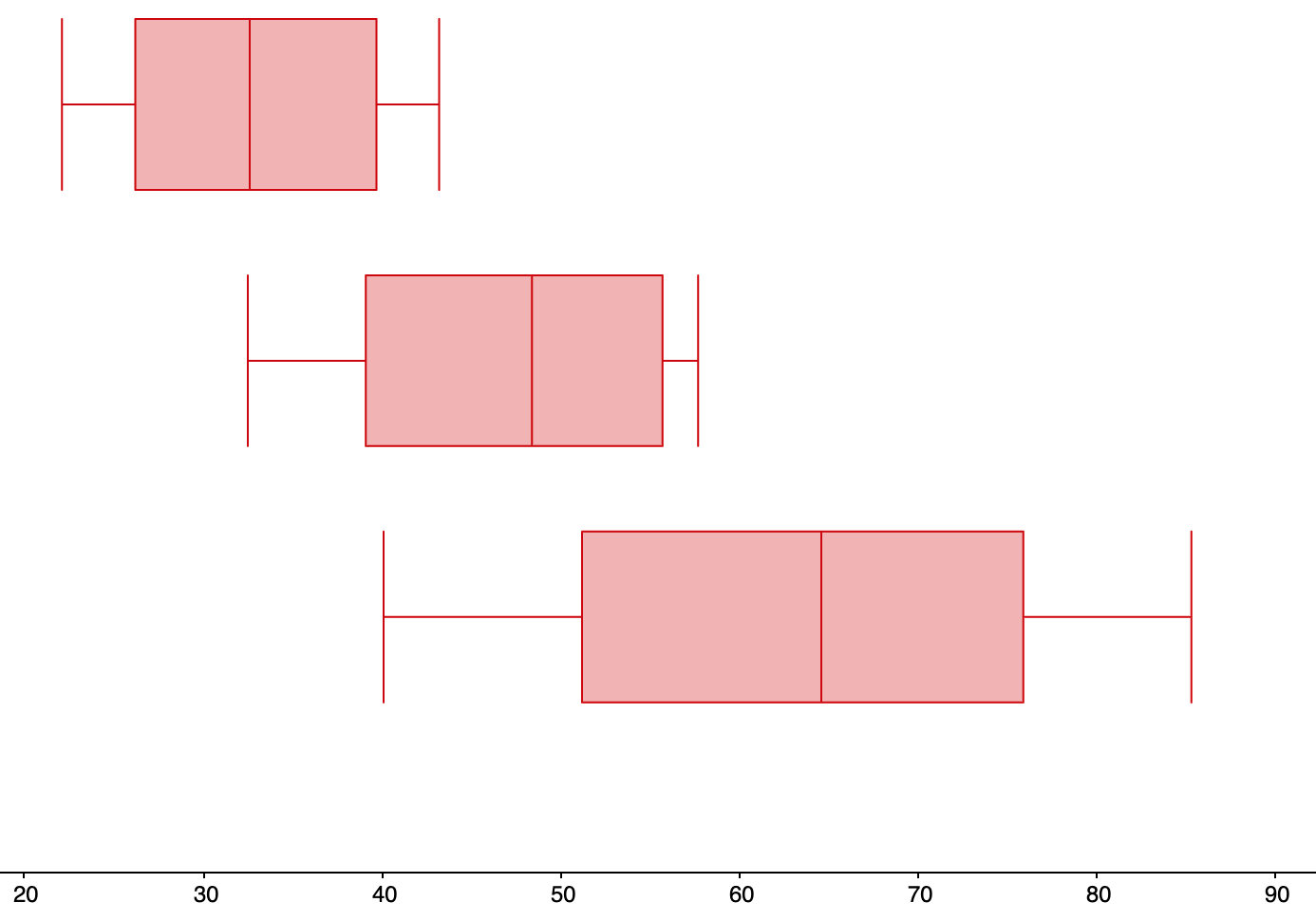
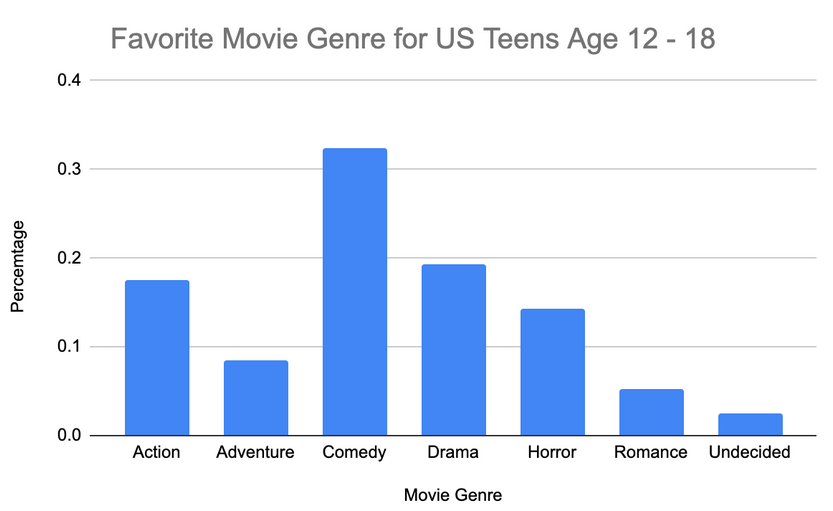
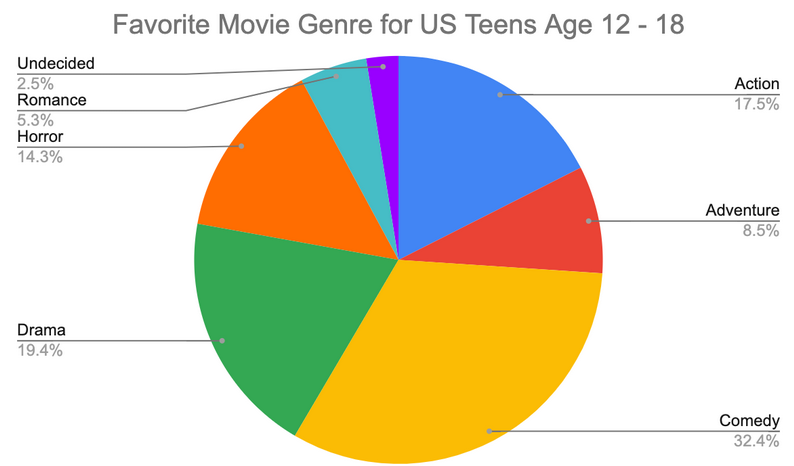
  5. Min Q1 Median Q3 Max
    $3.25 $3.75 $4.75 $5.00 $8.95
  6. \(\text{Range}=\$8.95-\$3.25=\$5.70\)
    \(\text{IQR}=\$5-\$3.75=\$1.25\)
  7. Boxplot using the 5-number summary above showing one outlier at $8.95

3.5.13.

Solution.
  1. The mean is about $26,200 and the standard deviation is about $10,000.
  2. The majority of cars sell for between $16,200 and $36,200.
  3. Min Q1 Median Q3 Max
    $12 thousand $18 thousand $26 thousand $32 thousand $45 thousand
  4. \(\text{Range} = 45 - 12 = 33\)
    \(\text{IQR}= 32 - 18 = 14\)
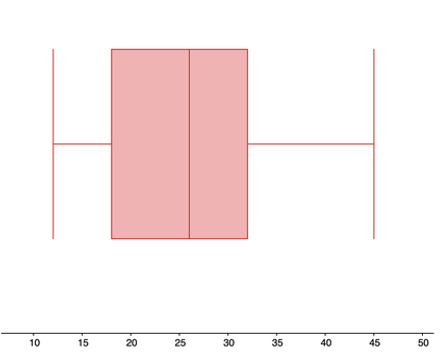
  5. A boxplot using the 5-number summary above; no outliers are shown

3.5.15.

Solution.
  1. \(\displaystyle z=\frac{(30.4-35)}{1.35}=\frac{-4.6}{1.35} \approx -3.41\)
  2. 30.4 mpg is 3.41 standard deviations below the mean so the car is getting unusually low gas mileage.

3.6 The Normal Distribution
3.6.8 Exercises

3.6.8.1.

Solution.
The shape of a Normal distribution is unimodal, symmetric and bell-shaped.

3.6.8.3.

Solution.
The median tree diameter is approximately 35 inches.

3.6.8.5.

Solution.
Approximately 68% of the values fall within one standard deviation of the mean.

3.6.8.7.

Solution.
Approximately 99.7% of the values fall within three standard deviations of the mean.

3.6.8.9.

Solution.
Approximately 27% of the values fall between the first and second standard deviations from the mean.

3.6.8.11.

Solution.
Approximately 4.7% of the values fall between the second and third standard deviations from the mean.

3.6.8.13.

Solution.
Approximately 34% of the values fall between the mean and one standard deviation below the mean.

3.6.8.15.

Solution.
  1. Here is the graph:
  2. Data values from 4.5 to 6.5 inches fall within one standard deviation of the mean.
  3. The percentage of data that fall between 3 and 10.5 inches is \(13.5\%+34\%+34\%+13.5\%+2.35\%=97.35\%\text{.}\)
  4. The percentage of data that fall below 1.5 inches is \(0.15\%\text{.}\)

3.6.8.17.

Solution.
  1. Here is the graph:
  2. The range of data values that fall within three standard deviations of the mean is $4 to $16.
  3. The percentage of data that lie between $6 and $14 is \(95\%\text{.}\)
  4. The percentage of data that lie above $14 is \(2.35\%+0.15\%=2.5\%\text{.}\)

3.6.8.19.

Solution.
  1. Here is the graph:
  2. The percentage of the data values that lie above 18.6 ounces is \(0.2302\) or \(23.028\%\text{.}\)
  3. The percentage of the data values that lie between 9 and 20.2 ounces is \(0.8076\) or \(80.76\%\text{.}\)
  4. The percentage of the data values that lie below 13.7 ounces is \(0.3341\) or \(33.41\%\text{.}\)

3.6.8.21.

Solution.
  1. Here is the graph:
  2. \(P(X \leq 82)=0.3341\text{.}\)
  3. \(P(76 \leq X \leq 90)=0.6632\text{.}\)
  4. \(P(X \geq 100)=0.0161\text{.}\)

3.6.8.23.

Solution.
A z-score measures how many standard deviations from the mean a data value is.

3.6.8.25.

Solution.
\(Z=\frac{6.2-6}{1.5}\approx=0.13\) standard deviations.

3.6.8.27.

Solution.
\(Z=\frac{5-10}{2}=-2.5\) standard deviations.

3.6.8.29.

Solution.
The confidence interval is \((\$2.20, \$2.50)\text{.}\) This means that we are 95% confident that the true average amount of change for all those who carry a purse is between $2.20 and $2.50.

3.6.8.31.

Solution.
The margin of error is 0.2156 cm. The confidence interval is approximately \((82.28, 84.72)\text{.}\) This means that we are 95% confident that the true population parameter is between approximately 82.28 and 84.72 cm.

4 Probability
4.1 Contingency Tables
4.1.10 Exercises

4.1.10.1.

Solution.
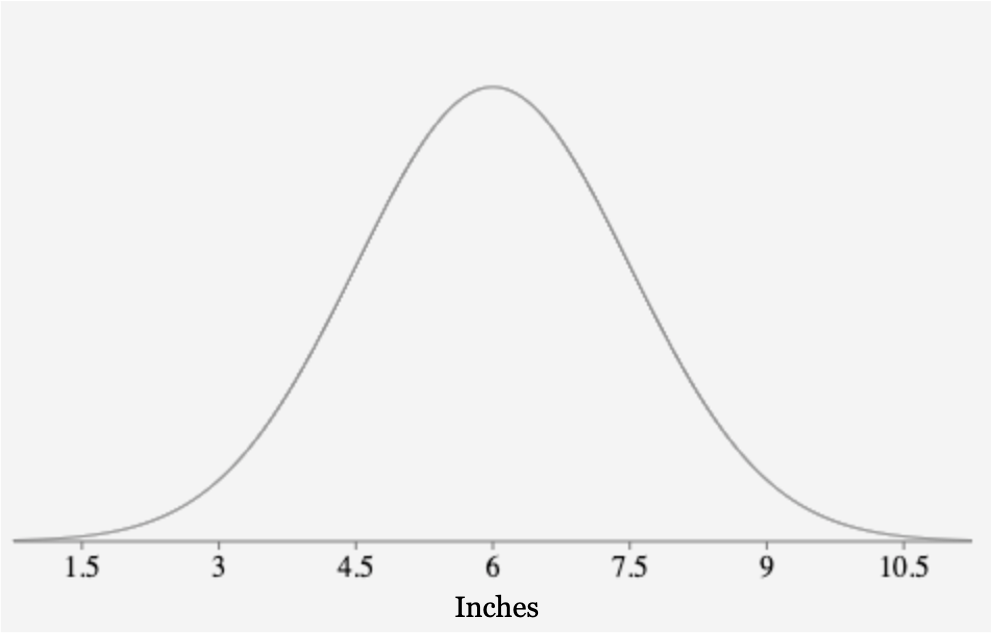
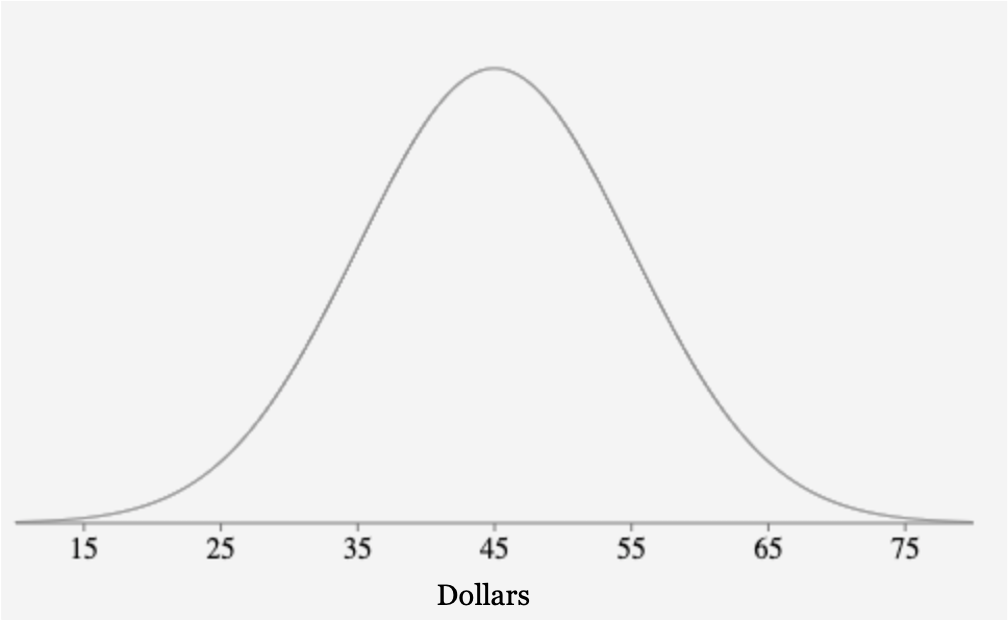
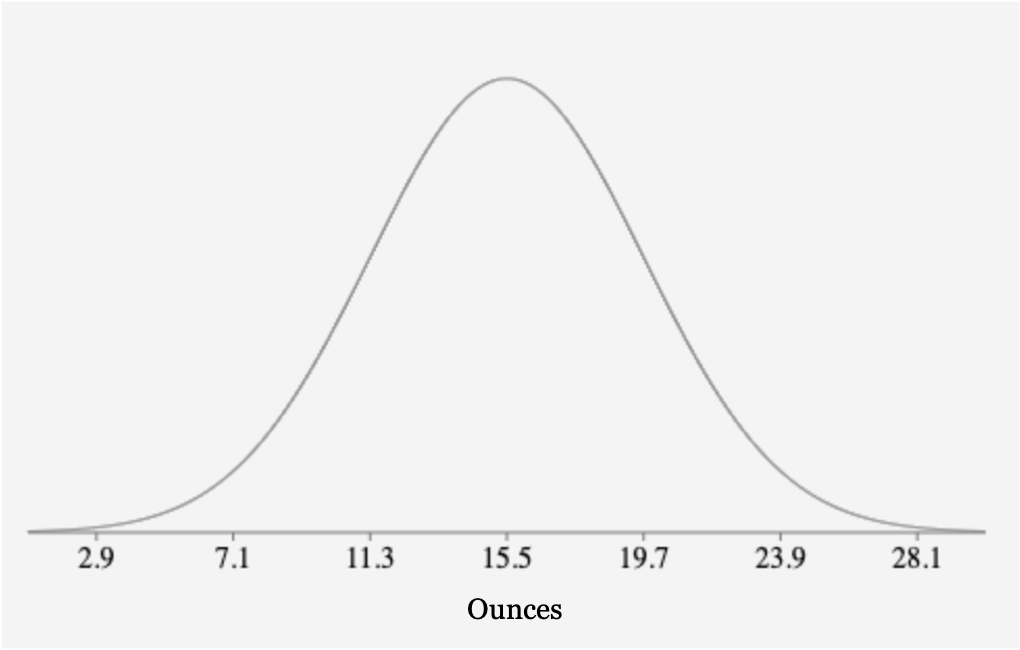
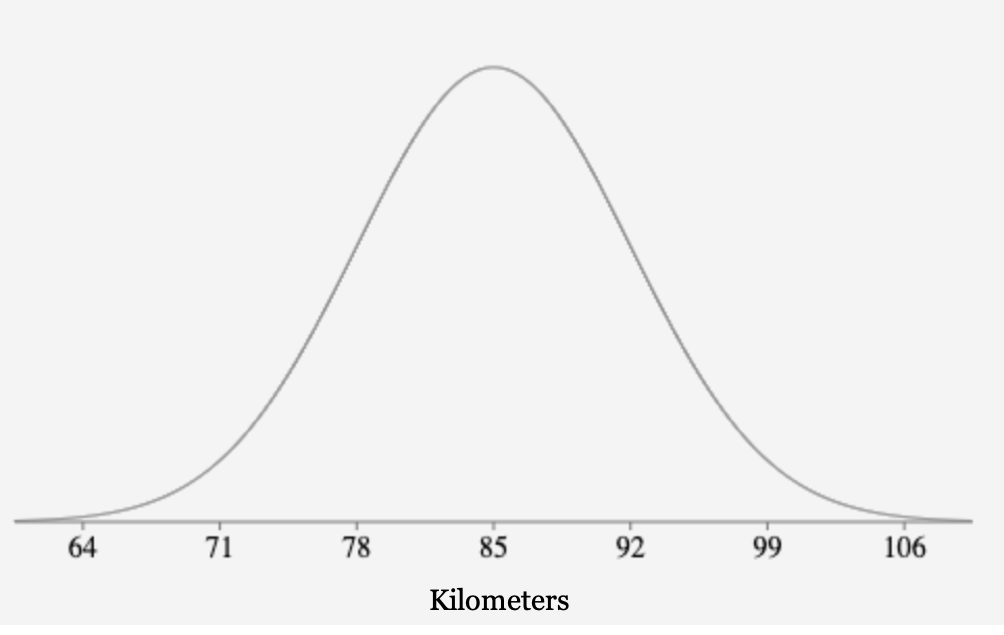
Food Insecure Not Food Insecure Total
Housing Insecure 380 60 440
Not Housing Insecure 300 460 760
Total 680 520 1200

4.1.10.3.

Solution.
Breakfast No Breakfast Total
Floss 12 49 61
No Floss 3 8 11
Total 15 57 72

4.1.10.5.

Solution.
A Not A Total
B 10 20 30
Not B 20 25 45
Total 30 45 75

4.1.10.7.

Solution.
  1. \begin{align*} \text{P(In morning class)}\amp=\frac{39}{65}\\ \amp= 0.60\text{ or } 60\% \end{align*}
  2. \begin{align*} \text{P(Earned a C)}\amp=\frac{25}{65}\\ \amp\approx 0.385 \text{ or } 38.5\% \end{align*}
  3. \begin{align*} \text{P(Earned an A and in afternoon class)}\amp=\frac{10}{65}\\ \amp\approx 0.154 \text{ or } 15.4\% \end{align*}
  4. \begin{align*} \text{P(Earned an A given in morning class)}\amp=\frac{8}{39}\\ \amp\approx 0.205 \text{ or } 20.5\% \end{align*}
  5. \begin{align*} \text{P(In morning class or earned B)}\amp=\frac{43}{65}\\ \amp\approx 0.662 \text{ or } 66.2\% \end{align*}

4.1.10.9.

Solution.
  1. \begin{align*} \text{P(no credit cards)}\amp=\frac{27}{81}\\ \amp\approx 0.333 \text{ or } 33.3\% \end{align*}
  2. \begin{align*} \text{P(one credit card)}\amp=\frac{15}{81}\\ \amp\approx 0.185 \text{ or } 18.5\% \end{align*}
  3. \begin{align*} \text{P(no credit cards and over age 35)}\amp=\frac{18}{81}\\ \amp\approx 0.222 \text{ or } 22.2\% \end{align*}
  4. \begin{align*} \text{P(between ages of 18 and 35,or have zero credit cards)}\amp=\frac{51}{81}\\ \amp\approx 0.630 \text{ or } 63.0\% \end{align*}
  5. \begin{align*} \text{P(no credit cards given between ages of 18 and 35)}\amp=\frac{9}{33}\\ \amp\approx 0.273 \text{ or } 27.3\% \end{align*}
  6. \begin{align*} \text{P(no credit cards given over age 35)}\amp=\frac{18}{48}\\ \amp=0.375 \text{ or } 37.5\% \end{align*}
  7. Yes, it appears that having no credit cards depends on age. The probability of having no credit cards for people over age 35 is significantly greater than the probability of having no credits for people between the ages of 18 and 35.

4.1.10.11.

Solution.
  1. \begin{align*} \text{P(not survive)}\amp=\frac{1490}{2201}\\ \amp\approx 0.677 \text{ or } 67.7\% \end{align*}
  2. \begin{align*} \text{P(crew)}\amp=\frac{885}{2201}\\ \amp\approx 0.402 \text{ or } 40.2\% \end{align*}
  3. \begin{align*} \text{P(first class and not survive)}\amp=\frac{122}{2201}\\ \amp\approx 0.055 \text{ or } 5.5\% \end{align*}
  4. \begin{align*} \text{P(not survive or crew)}\amp=\frac{1702}{2201}\\ \amp\approx 0.773 \text{ or } 77.3\% \end{align*}
  5. \begin{align*} \text{P(survived given first class)}\amp=\frac{203}{325}\\ \amp\approx 0.625 \text{ or } 62.5\% \end{align*}
  6. \begin{align*} \text{P(survived given second class)}\amp=\frac{118}{285}\\ \amp\approx 0.414 \text{ or } 41.4\% \end{align*}
  7. \begin{align*} \text{P(survived given third class)}\amp=\frac{178}{706}\\ \amp\approx 0.252 \text{ or } 25.2\% \end{align*}
  8. Yes, it does appear that survival depended on the passenger’s class. The probability of survival for first class passengers is significantly greater than the probability of survival for second class passengers and is more than double the probability of survival for third class passengers.

4.1.10.13.

Solution.
  1. Game/Software No Game/Software Total
    Computer 10% 5% 15%
    No Computer 15% 70% 85%
    Total 25% 75% 100%
  2. \begin{align*} \text{P(no computer and no game/software)}\amp=\frac{70\%}{100\%}\\ \amp=0.7 \text{ or } 70\% \end{align*}
  3. \begin{align*} \text{P(computer or game/software)}\amp=\frac{30\%}{100\%}\\ \amp=0.3 \text{ or } 30\% \end{align*}
  4. \begin{align*} \text{P(game/software given computer)}\amp=\frac{10\%}{15\%}\\ \amp\approx 0.667 \text{ or } 66.7\% \end{align*}
  5. \begin{align*} \text{P(game/software given no computer)}\amp=\frac{15\%}{85\%}\\ \amp\approx 0.176 \text{ or } 17.6\% \end{align*}
  6. Purchasing a game/software and purchasing a computer appear to be depended. The probability of purchasing a game/software for computer buyers was almost 50% greater than the probability of purchasing a game/software among customers who did not purchase a computer.

4.1.10.15.

Solution.
  1. Hardcover Paperback Total
    Fiction 13 59 72
    Nonfiction 15 8 23
    Total 28 67 95
  2. \begin{align*} \text{P(non-fiction and paperback)}\amp=\frac{8}{95}\\ \amp\approx 0.084 \text{ or } 8.4\% \end{align*}
  3. \begin{align*} \text{P(fiction given hardcover)}\amp=\frac{13}{28}\\ \amp\approx 0.464 \text{ or } 46.4\% \end{align*}

4.2 Theoretical Probability
4.2.15 Exercises

4.2.15.1.

Solution.
  1. \(\displaystyle 2/13\)
  2. \(\displaystyle 6/13\)
  3. \(\displaystyle 0\)
  4. \(\displaystyle 25/169\)
  5. \(\displaystyle 1/13\)

4.2.15.3.

Solution.
  1. \(\displaystyle 11/12\)
  2. \(\displaystyle 1/6\)

4.2.15.5.

Solution.
\(6241/10000\)

4.2.15.7.

Solution.
  1. \(\displaystyle 1/8\)
  2. \(\displaystyle 1/8\)

4.2.15.9.

Solution.
  1. \(\displaystyle 1/4\)
  2. \(\displaystyle 1/6\)
  3. \(\displaystyle 1/9\)

4.2.15.11.

Solution.
  1. \(\displaystyle 4/52=1/13\)
  2. \(\displaystyle 13/52=1/4\)
  3. \(\displaystyle 26/52=1/2\)
  4. \(\displaystyle 16/52=4/13\)

4.2.15.13.

Solution.
\(60/1320=1/22 \approx 0.045\) or 4.5%

4.2.15.15.

Solution.
\(1 - \text{P(no blue marbles) }=1110/1320=37/44 \approx 0.841\) or 84.1%

4.3 Expected Value
4.3.2 Exercises

4.3.2.1.

Solution.
  1. Die roll Gold Silver Black
    Outcome $3 $2 -$1>
    Probability \(3/37\) \(6/37\) \(28/37\)
  2. \(3(3/37)+2(6/37)-1(28/37)= -0.19\)
    The expected value is approximately -$0.19. That is, you would lose about $0.19 on average each time you pick a marble.

4.3.2.3.

Solution.
  1. Die roll outcome 1, 2, 3, or 4 5 6
    Outcome $5 $0 -$2
    Probability \(1/6\) \(1/6\) \(4/6\)
  2. \(5(1/6)+0(1/6)-2(4/6)=-0.50\)
    The expected value is about -$0.50 which means you would lose 50 cents on average each time you roll the die.
  3. No, you should not play this game (unless you want to give your friend your money.

4.3.2.5.

Solution.
The company’s expected profit is $45.55 per warranty sold.

4.3.2.7.

Solution.
The company’s expected value on each policy is $22 which means they will make $22, on average, per policy sold.

4.3.2.9.

Solution.
The expected value for this raffle is -$3.

4.3.2.11.

Solution.
The expected value for this game is approximately -$6.

4.3.2.13.

Solution.
Answers will vary since you are making up your own problem.

4.4 Chapter 4 Review

4.4.1.

Solution.
  1. P(in the afternoon class) \(\approx 28/60=0.4667\)
  2. P(earned an A) \(25/60 \approx 0.4167\)
  3. P(earned a B and was in the afternoon class) \(= 13/60 \approx 0.2167\)
  4. P(earned a C given the student was in the morning class) \(=7/32 \approx 0.2188\)
  5. P(is in the morning class given that the student earned a B) \(=11/24 \approx 0.4583\)

4.4.3.

Solution.
Type Channel 2 Channel 6 Channel 8 Channel 12 Total
Drama 5 2 4 4 15
Sitcom 6 9 7 3 25
Game Show 4 4 3 4 15
News 3 2 2 3 10
Total 18 17 16 14 65
  1. P(Sitcom or Game Show) \(=40/65 \approx 0.6154\)
  2. P(Drama and Channel 8) \(=4/65 \approx 0.0615\)
  3. P(Channel 8 or Channel 2) \(=34/65 \approx 0.5231\)
  4. P(Drama given that it is on Channel 6) \(=2/17 \approx 0.1176\)
  5. P(Channel 12 given that it’s a sitcom) \(=3/25=0.12\)
  6. P(Game show given that it is on Channel 2) \(=4/18 \approx 0.2222\)

4.4.5.

Solution.
  1. \(\displaystyle \text{P(red)}=12/25=0.48\)
  2. \(\displaystyle \text{P(not white)}=17/25=0.68\)
  3. \(\displaystyle \text{P(Yellow or Red)}=17/25=0.68\)
  4. \(\displaystyle \text{P(Blue)}=0/25=0\)
  5. \(\displaystyle \text{P(Two reds)}=144/625=0.2304\)
  6. \(\displaystyle \text{P(Red then yellow)}=60/600=0.10\)

4.4.7.

Solution.
  1. \(\displaystyle \text{P(Four heads)}=0.0625\)
  2. \(\displaystyle \text{P(No heads)}=0.0625\)

4.4.9.

Solution.
  1. \(\displaystyle \text{P(Both too little)} \approx 0.4624\)
  2. \(\displaystyle \text{P(Neither too little)} \approx 0.1024\)

4.4.11.

Solution.
  1. Color Black Orange Yellow
    x $3 $2 -$1
    P(x) \(2/26\) \(4/26\) \(20/26\)
  2. The expected value is -$0.23.
  3. No, you should not play this game because it has a negative expected value.

4.4.13.

Solution.
Outcome Product Failed Didn’t Fail
x -$450 $0
P(x) 0.015 0.985
The expected loss per warranty is $48.25. (Note that if you include the $55.00 a person pays for a warranty, the expected profit will be $6.75 per warranty)

5 Democracy
5.1 Apportionment
5.1.11 Exercises

5.1.11.1.

Solution.
  1. Math: 6 tutors, English: 5 tutors, Chemistry: 3 tutors, Biology: 1 tutor
  2. Math: 7 tutors, English: 5 tutors, Chemistry: 2 tutors, Biology: 1 tutor, Modified divisor 47
  3. Math: 6 tutors, English: 5 tutors, Chemistry: 3 tutors, Biology: 1 tutor, Modified divisor 52
  4. Math: 6 tutors, English: 5 tutors, Chemistry: 3 tutors, Biology: 1 tutor, Divisor 53

5.1.11.3.

Solution.
  1. Morning: 1 salesperson, Midday: 5 salespeople, Afternoon: 6 salespeople, Evening: 8 salespeople
  2. Morning: 1 salesperson, Midday: 4 salespeople, Afternoon: 7 salespeople, Evening: 8 salespeople, Modified divisor 62
  3. Morning: 1 salesperson, Midday: 5 salespeople, Afternoon: 6 salespeople, Evening: 8 salespeople, Divisor 67.5
  4. Morning: 1 salesperson, Midday: 5 salespeople, Afternoon: 6 salespeople, Evening: 8 salespeople, Divisor 67.5

5.1.11.5.

Solution.
  1. Aisha: 18 coins, Basir: 14 coins, Carlos: 4 coins
  2. Aisha: 19 coins, Basir: 14 coins, Carlos: 3 coins, Modified divisor 400
  3. Aisha: 19 coins, Basir: 14 coins, Carlos: 3 coins, Modified divisor 410
  4. Aisha: 19 coins, Basir: 14 coins, Carlos: 3 coins, Modified divisor 410

5.1.11.7.

Solution.
  1. A: 5 seats, B: 79 seats, C: 20 seats, D: 12 seats
  2. A: 4 seats, B: 80 seats, C: 20 seats, D: 12 seats, Modified divisor 6950
  3. A: 5 seats, B: 78 seats, C: 20 seats, D: 13 seats, Modified divisor 7128
  4. A: 5 seats, B: 78 seats, C: 20 seats, D: 13 seats, Modified divisor 6950

5.1.11.9.

Solution.
  1. A: 40 seats, B: 24 seats, C: 15 seats, D: 30 seats, E: 10 seats
  2. A: 41 seats, B: 24 seats, C: 14 seats, D: 30 seats, E: 10 seats, Modified divisor 19,700
  3. A: 40 seats, B: 24 seats, C: 15 seats, D: 30 seats, E: 10 seats, Modified divisor 20,100
  4. A: 40 seats, B: 24 seats, C: 15 seats, D: 30 seats, E: 10 seats, Modified divisor 20,125

5.1.11.11.

Solution.
  1. A: 23 seats, B: 16 seats, C: 77 seats, D: 30 seats, E: 21 seats, F: 33 seats
  2. A: 22 seats, B: 16 seats, C: 78 seats, D: 30 seats, E: 21 seats, F: 33 seats, Modified divisor 148.5
  3. A: 23 seats, B: 16 seats, C: 77 seats, D: 30 seats, E: 21 seats, F: 33 seats, Divisor 150
  4. A: 23 seats, B: 16 seats, C: 77 seats, D: 30 seats, E: 21 seats, F: 33 seats, Divisor 150

5.1.11.13.

Solution.
  1. . A: 19 seats, B: 19 seats, C: 22 seats, D: 22 seats, E: 81 seats, F: 87 seats
  2. A: 28 seats, B: 19 seats, C: 22 seats, D: 22 seats, E: 82 seats, F: 87 seats, Modified divisor 4347
  3. A: 19 seats, B: 19 seats, C: 22 seats, D: 22 seats, E: 81 seats, F: 87 seats, Divisor 4400.4
  4. A: 19 seats, B: 19 seats, C: 22 seats, D: 22 seats, E: 81 seats, F: 87 seats, Divisor 4400.4

5.1.11.15.

Solution.
  1. A: 4 seats, B: 4 seats, C: 2 seats
  2. It is not possible to assign 11 seats with Hamilton’s method in this case.
  3. States A and C are the same size, so they have the same decimal value. They would both get an additional seat at the same time but there is only one seat to give. Answers will vary on fair solutions.
  4. Yes, with a modified divisor of 1200 we get A: 5 seats, B: 5 seats and C: 1 seat.

5.1.11.17.

Solution.
2010: Douglass: 8, Parks: 4, King: 10, Du Bois: 11, Lewis: 17
2020: Douglass: 8, Parks: 5, King: 10, Du Bois: 11, Lewis: 16
The populations in King and Lewis counties grew, but Parks got an extra seat from Lewis. This doesn’t seem fair.

5.1.11.19.

Solution.
  1. Clatsop: 2 counselors, Siletz: 11 counselors
  2. The divisor was 698.38, so 4 new guidance counselors should be hired for Cayuse.
  3. Clatsop: 3 counselors, Siletz: 10 counselors, Cayuse: 4 counselors
  4. Cayuse did get 4 counselors, but one of the counselors from Siletz went to Clatsop. That doesn’t seem fair because their populations didn’t change.

5.2 Voting Methods
5.2.12 Exercises

5.2.12.1.

Solution.
Number of voters 3 3 1 3 2
1st choice A A B B C
2nd choice B C A C A
3rd choice C B C A B

5.2.12.3.

Solution.
  1. There are 47 voters.
  2. A majority is 24 votes.
  3. Atlanta wins the plurality method with 19 votes.
  4. Buffalo wins the Instant Runoff Method with 28 votes.
  5. The points are: Atlanta 94, Buffalo 111 and Chicago 77. Buffalo wins the Borda Count Method.
  6. The points are: Buffalo 2, Atlanta 1. Buffalo wins with Copeland’s method.

5.2.12.5.

Solution.
  1. There are 12 voters.
  2. A majority is 7 votes.
  3. Biology wins the plurality method with 5 votes.
  4. Biology wins the Instant Runoff Method with 7 votes.
  5. The points are: Art 22, Biology 26 and Calculus 24. Biology wins the Borda Count Method.
  6. The points are: Biology 2, Calculus 1. Biology wins with Copeland’s method.

5.2.12.7.

Solution.
  1. There are 31 votes.
  2. A majority is 16 votes.
  3. F wins the plurality method with 12 votes.
  4. E wins the Instant Runoff Method with 16 votes.
  5. The points are: D 66, E 59 and F 61. D wins the Borda Count Method.
  6. The points are: D 2, E 1. D wins with Copeland’s method.

5.2.12.9.

Solution.
  1. There are 460 voters.
  2. A majority is 231 votes.
  3. A wins the plurality method with 150 votes.
  4. A wins the Instant Runoff Method with 290 votes.
  5. The points are: A 1140, B 1060, C 1160 and D 1240. D wins the Borda Count method.
  6. The points are: A 1, B 1, C 2, D 2. C and D tie with Copeland’s method.

5.2.12.11.

Solution.
  1. There are 92 voters.
  2. A majority is 47 votes.
  3. K wins the plurality method with 38 votes.
  4. K wins the Instant Runoff Method with 54 votes.
  5. The points are: I 273, J 152, K 267 and L 228. I wins the Borda Count Method.
  6. The points are: I 2, K 3, L2. K wins with Copeland’s method.

5.2.12.13.

Solution.
  1. There are 90 voters.
  2. A majority is 46 votes.
  3. Q wins the plurality method with 26 votes.
  4. S wins the Instant Runoff Method with 50 votes.
  5. The points are: Q 250, R 201, S 243 and T 206. Q wins the Borda Count Method.
  6. The points are: Q 2, R 1 and S 3. S wins with Copeland’s method.

5.2.12.15.

Solution.
  1. There are 107 voters.
  2. A majority is 54 votes.
  3. E wins the plurality method with 39 votes.
  4. B wins the Instant Runoff Method with 54 votes.
  5. The points are: A 357, B 398, C 305, D 219, E 326. B wins the Borda Count Method.
  6. The points are: A 2, B 4, C 2, D 1, E 1. B wins with Copeland’s method.

5.2.12.17.

Solution.
  1. There are 127 voters.
  2. A majority is 64 votes.
  3. K wins the plurality method with 35 votes.
  4. M wins the Instant Runoff Method with 79 votes.
  5. The points are: K 430, L 402, M 376, N 375, O 322. K wins the Borda Count Method.
  6. The points are: K 4, L 2, M 2, N 2. K wins with Copeland’s method.

5.3 The Popular Vote, Electoral College and Electoral Power
5.3.4 Exercises

5.3.4.1.

Solution.
The president is elected through a process called The Electoral College. States send a certain number of electors, based on their populations, and whichever candidate gets the most votes from these electors becomes president.

5.3.4.3.

Solution.
Two senators represent each state.

5.3.4.5.

Solution.
There are 435 Representatives in the U.S. House of Representatives.

5.3.4.7.

Solution.
  1. State Population Number of
    Representatives    		 Number of
    Senators    		 Number of
    Electors
    Gandhi 450,000 9 2 11
    Mandela 150,000 3 2 5
    Gbowee 600,000 12 2 14
    Total 1,200,000 24 6 30
    This state has 30 electors.
  2. A majority of electoral votes would be 16 votes.

5.3.4.9.

Solution.
  1. State Population Number of
    Representatives    		 Number of
    Senators    		 Number of
    Electors
    Tamez 280,000 7 2 9
    Teters 200,000 5 2 7
    Herrington 400,000 10 2 12
    Osawa 360,000 9 2 11
    Total 1,240,000 31 8 39
    This state has 39 electors.
  2. A majority of electoral votes would be 20 electoral votes.

5.3.4.11.

Solution.
  1. State Votes for
    Candidate A    		 Votes for
    Candidate B    		 Number of
    Electoral
    Votes for A    		 Number of
    Electoral
    Votes for B
    Gandhi 216,000 234,000 0 11
    Mandela 37,500 112,500 0 5
    Gbowee 489,450 110,550 14 0
    Total Votes 742,950 457,050 14 16
    A wins the popular vote with 61.9% of the votes.
  2. B wins the electoral college and becomes the president with 53.5% of the electoral votes.

5.3.4.13.

Solution.
  1. State Votes for
    Candidate A    		 Votes for
    Candidate B    		 Number of
    Electoral
    Votes for A    		 Number of
    Electoral
    Votes for B
    Tamez 95,480 184,250 0 9
    Teters 104,200 95,800 7 0
    Herrington 203,600 196,400 12 0
    Osawa 46,080 313,920 0 11
    Total Votes 449,360 790,640 19 20
    B wins the popular vote with 63.6% of the vote.
  2. B wins the electoral college and becomes the president with 51% of the electoral votes.

5.3.4.15.

Solution.
  1. State Population Number
    of
    Representatives    		 Number
    of
    Senators    		 Number
    of
    Electors    		 Electoral
    Votes per
    50,000 people
    Gandhi 450,000 9 2 11 1.22
    Mandela 150,000 3 2 5 1.67
    Gbowee 600,000 12 2 14 1.17
    The state of Mandela has the most electoral power.
  2. The state of Gbowee has the least electoral power.

5.3.4.17.

Solution.
  1. State Population Number
    of
    Representatives    		 Number
    of
    Senators    		 Number
    of
    Electors    		 Electoral
    Votes per
    50,000 people
    Tamez 280,000 7 2 9 1.29
    Teters 200,000 5 2 7 1.40
    Herrington 400,000 10 2 12 1.20
    Osawa 360,000 9 2 11 1.22
    The state of Teters has the most electoral power.
  2. The state of Herrington has the least electoral power.

5.3.4.19.

Solution.
Possible combinations are Gandhi/Mandela/Gbowee, Gandhi/Mandela, Mandela/Gbowee, or Gandhi/Gbowee. The minimum number of votes needed is 300,002.

5.3.4.21.

Solution.
Possible combinations are Tamez/Teters/Herrington/Osawa, Tamez/Teters/Herrington, Tamez/Teters/Osawa, Tamez/Herrington/Osawa, Teters/Herrington/Osawa, Tamez/Herrington, Tamez/Osawa, or Herrington/Osawa. The minimum number of votes needed is 320,002.

5.4 Gerrymandering and How to Measure It
5.4.5 Exercises

5.4.5.1.

Solution.
Redistricting happens every 10 years after the census is completed. A state might not change its districts unless the number of seats in the U.S. House of Representatives has changed.

5.4.5.3.

Solution.
The rules are districts must be contiguous and you can’t gerrymander by race.

5.4.5.5.

Solution.
The most proportional representation would be 4 Republican seats and 3 Democratic seats.

5.4.5.7.

Solution.
The most proportional representation would be 2 Republican seats and 1 Democratic seat.

5.4.5.9.

Solution.
The most proportional representation would be 6 Republican seats, 4 Democratic seats and 1 Green Party seat.

5.4.5.11.

Solution.
  1. A majority is 4 votes.
  2. The Democrats won 2 seats and the Republicans won 2 seats.
  3. The efficiency gap is \(6/28= 21.43\%\)
    District D Votes R Votes D Surplus Votes R Surplus Vote
    1 1 6 1 \(6-4=2\)
    2 3 4 3 \(4-4=0\)
    3 6 1 \(6-4=2\) 1
    4 7 0 \(7-4=3\) 0
    Total 17 11 9 3
  4. Each seat is worth 25% of the voters.
  5. The efficiency gap is worth less than one seat (0.86 seats).
  6. This map is ok because the efficiency gap is less than one seat. It should either be D3, R1 or D2, R2.

5.4.5.13.

Solution.
  1. A majority is 3 votes.
  2. The Democrats won 1 seat and the Republicans won 4 seats.
  3. The efficiency gap is \(8/25= 32\%\)
    District D Votes R Votes D Surplus Votes R Surplus Vote
    1 2 3 2 \(3-3=0\)
    2 2 3 2 \(3-3=0\)
    3 2 3 2 \(3-3=0\)
    4 4 1 \(4-3=1\) 1
    5 2 3 2 \(3-3=0\)
    Total 12 13 9 1
  4. Each seat is worth 20% of the voters.
  5. The efficiency gap is worth 1.6 seats.
  6. This map is not fair because the efficiency gap is more than one seat. A fairer map would be D3, R2 or D2, R3.

5.4.5.15.

Solution.
  1. A majority is 5 votes.
  2. The Democrats won 2 seats and the Republicans won 3 seats.
  3. The efficiency gap is \(4/45= 8.89\%\)
    District D Votes R Votes D Surplus Votes R Surplus Vote
    1 2 7 2 \(7-5=2\)
    2 5 4 \(5-5=0\) 4
    3 2 7 2 \(7-5=2\)
    4 5 4 \(5-5=0\) 4
    5 4 5 4 \(5-5=0\)
    Total 18 27 8 12
  4. Each seat is worth 20% of the voters.
  5. The efficiency gap is worth less than one seat (0.44).
  6. This map is fair because the efficiency gap is around 8% and the representation is exactly proportional to the population.

5.4.5.17.

Solution.
  1. A majority is 3 votes.
  2. The Democrats won 5 seats and the Republicans won 1 seat.
  3. The efficiency gap is \(12/30= 40\%\)
    District D Votes R Votes D Surplus Votes R Surplus Vote
    1 3 2 \(3-3=0\) 2
    2 3 2 \(3-3=0\) 2
    3 3 2 \(3-3=0\) 2
    4 3 2 \(3-3=0\) 2
    5 3 2 \(3-3=0\) 2
    6 0 5 0 \(5-3=2\)
    Total 15 15 0 12
  4. Each seat is worth 16.67% of the voters.
  5. The efficiency gap is worth 2.4 seats.
  6. This map is not fair because the efficiency gap is more than one seat. A more fair map would be D3, R3 because the population is evenly split.

5.4.5.19.

Solution.
  1. A majority is 5 votes.
  2. The Democrats won 1 seat and the Republicans won 5 seats.
  3. The efficiency gap is \(6/54= 11.11\%\)
    District D Votes R Votes D Surplus Votes R Surplus Vote
    1 2 7 2 \(7-5=2\)
    2 2 7 2 \(7-5=2\)
    3 9 0 \(9-5=4\) 0
    4 2 7 2 \(7-5=2\)
    5 1 8 1 \(8-5=3\)
    6 4 5 4 \(5-5=0\)
    Total 20 34 15 9
  4. Each seat is worth 16.67% of the voters.
  5. The efficiency gap is worth less than 1 seat. (0.67)
  6. This map is not fair because even though the efficiency gap is less than one seat, The Democrats should have at least 2 seats. A more fair map would be D2, R4.

5.4.5.21.

Solution.
  1. The most seats that can be won by the Democrats is 4 seats.
  2. The most seats that can be won by the Republicans is 4 seats.

5.4.5.23.

Solution.
  1. The most seats that can be won by the Democrats is 4 seats.
  2. The most seats that can be won by the Republicans is 5 seats.

5.5 Chapter 5 Review

5.5.1.

Solution.
  1. A: 10 seats, B: 17 seats, C: 12 seats, D: 39 seats
  2. A: 10 seats, B: 16 seats, C: 12 seats, D: 40 seats, Modified divisor 9,600
  3. A: 10 seats, B: 17 seats, C: 12 seats, D: 39 seats, Divisor 9,793.6
  4. A: 10 seats, B: 17 seats, C: 12 seats, D: 39 seats, Divisor 9,793.6

5.5.3.

Solution.
  1. A: 46 seats, B: 18 seats, C: 7 seats, D: 21 seats, E: 8 seats
  2. A: 47 seats, B: 18 seats, C: 7 seats, D: 20 seats, E: 8 seats, Modified divisor 12,400
  3. A: 47 seats, B: 18 seats, C: 7 seats, D: 20 seats, E: 8 seats, Modified divisor 12,555
  4. A: 47 seats, B: 18 seats, C: 7 seats, D: 20 seats, E: 8 seats, Modified divisor 12,555

5.5.5.

Solution.
  1. There are 134 voters.
  2. A majority is 68 votes.
  3. H wins the plurality method with 35 votes.
  4. F wins the Instant Runoff Method with 71 votes.
  5. The points are: E 275, F 364, G 372 and H 329. G wins in the Borda count method.
  6. The points are: F 3, G 2, H 1. F wins with Copeland’s method.

5.5.7.

Solution.
  1. There are 166 voters.
  2. A majority is 84 votes.
  3. N wins the plurality method with 37 votes.
  4. P wins the Instant Runoff Method with 101 votes.
  5. The points are: M 486, N 482, O 508, P 590, Q 424. P wins the Borda count method.
  6. The points are: M 1, N 2, O 3, P 4. P wins with Copeland’s method.

5.5.9.

Solution.
  1. State Population Number of
    Representatives    		 Number of
    Senators    		 Number of
    Electors
    Fonville 825,000 15 2 17
    Gurley 550,000 10 2 12
    Nevarez 275,000 5 2 7
    Total 1,650,000 30 6 36
    This state has 36 electors.
  2. A majority of electoral votes would be 19 votes.

5.5.11.

Solution.
  1. State Votes for
    Candidate A    		 Votes for
    Candidate B    		 Number of
    Electoral
    Votes for A    		 Number of
    Electoral
    Votes for B
    Fonville 684,750 140,250 17 0
    Gurley 257,400 292,600 0 12
    Nevarez 132,275 142,725 0 7
    Total Votes 1,074,425 575,575 17 19
    A wins the popular vote with 65.1% of the votes.
  2. B wins the electoral college and becomes the president with 52.8% of the electoral votes.

5.5.13.

Solution.
  1. State Population Number
    of
    Representatives    		 Number
    of
    Senators    		 Number
    of
    Electors    		 Electoral
    Votes per
    55,000 people
    Fonville 825,000 15 2 17 1.13
    Gurley 550,000 10 2 12 1.20
    Nevarez 275,000 5 2 7 1.40
    The state of Nevarez has the most electoral power.
  2. The state of Fonville has the least electoral power.

5.5.15.

Solution.
  1. A majority is 5 votes.
  2. The Democrats won 1 seat and the Republicans won 4 seats.
  3. The efficiency gap is \(20/45= 44.4\%\)
    District D Votes R Votes D Surplus Votes R Surplus Vote
    1 4 5 4 \(5-5=0\)
    2 4 5 4 \(5-5=0\)
    3 9 0 \(9-5=4\) 0
    4 4 5 4 \(5-5=0\)
    5 4 5 4 \(5-5=0\)
    Total 25 20 20 0
  4. Each seat is worth 20% of the voters.
  5. The efficiency gap is worth 2.2 seats.
  6. This map is not fair because the efficiency gap is more than two seats. A more fair map would be D3, R2.

5.6 Federal Budget, Deficit and National Debt
5.6.8 Exercises

5.6.8.1.

Solution.
Federal income comes from individual income taxes and business income taxes.

5.6.8.3.

Solution.
Twelve appropriations bills must be passed to approve the new federal budget.

5.6.8.5.

Solution.
A deficit is a shortfall in a single year and debt is the total of all the money owed.

5.6.8.7.

Solution.
  1. 4.3 billion
  2. 12.567 trillion
  3. 500 million or 0.5 billion
  4. 6.04 million

5.6.8.9.

Solution.
  1. 5,700,000
  2. 9,220,000,000,000
  3. 100,200,000,000
  4. 250,000,000,000

5.6.8.11.

Solution.
  1. The debt to GDP ratio for Columbia is approximately 55.13%.
  2. The amount of debt owed per person in Columbia is approximately C$ 5.541 million.
  3. The amount of interest paid per year per person in Columbia is approximately C$ 0.34 million or C$ 340,040.

5.6.8.13.

Solution.
  1. The debt to GDP ratio for Poland is approximately 61.74%.
  2. The amount of debt owed per person in Poland is approximately 31,773 zł.
  3. The amount of interest paid per year per person in Pakistan is approximately 1.36 zł.

5.6.8.15.

Solution.
  1. The debt to GDP ratio for South Africa is approximately 60.39%.
  2. The amount of debt owed per person in South Africa is approximately R. 68,550.
  3. The amount of interest paid per year per person in South Africa is approximately R. 3,612.

5.6.8.17.

Solution.
  1. Approximately $7.668 billion is budgeted for transportation.
  2. Approximately $2,255 is budgeted for health and human resources per person.
  3. Approximately $3.834 billion is budgeted for public safety in total.
PrevTopNext